-
摘要:
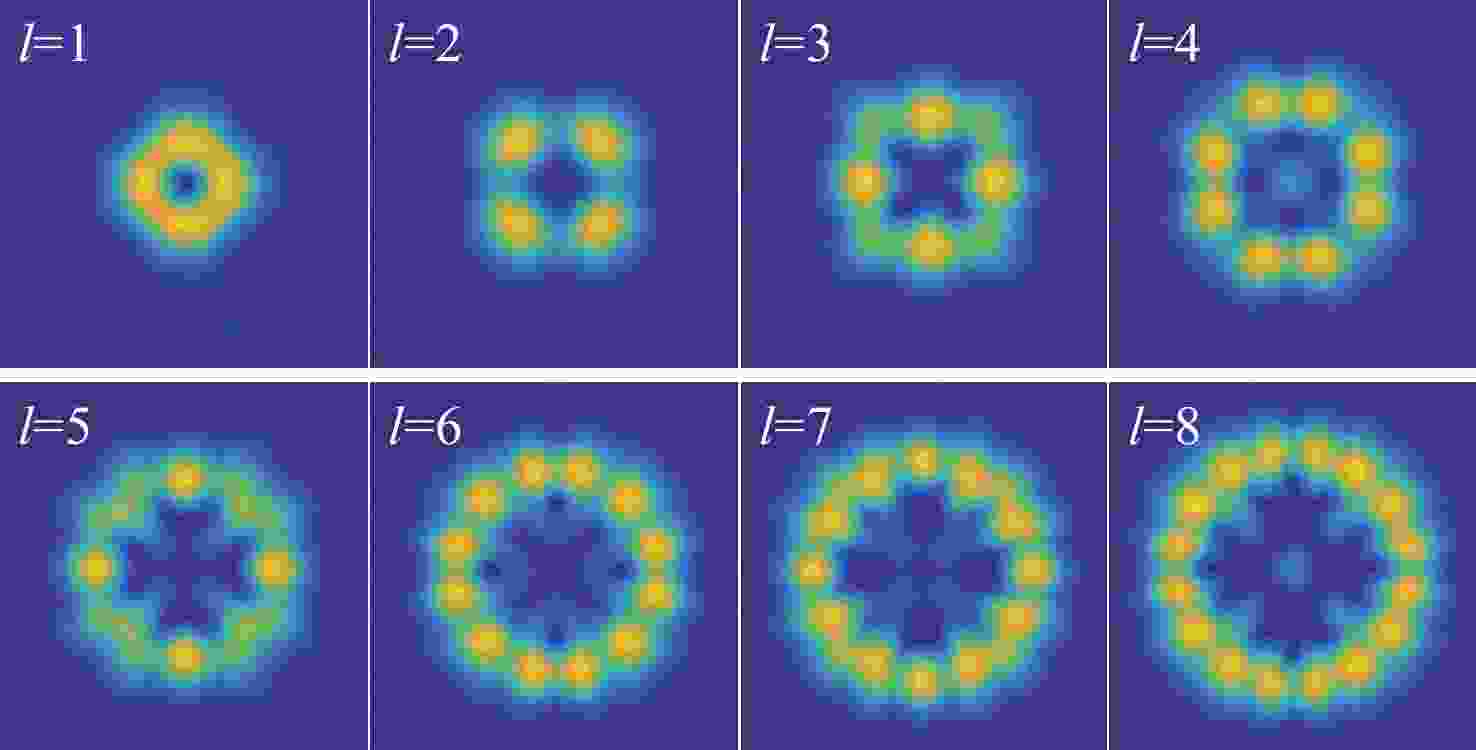
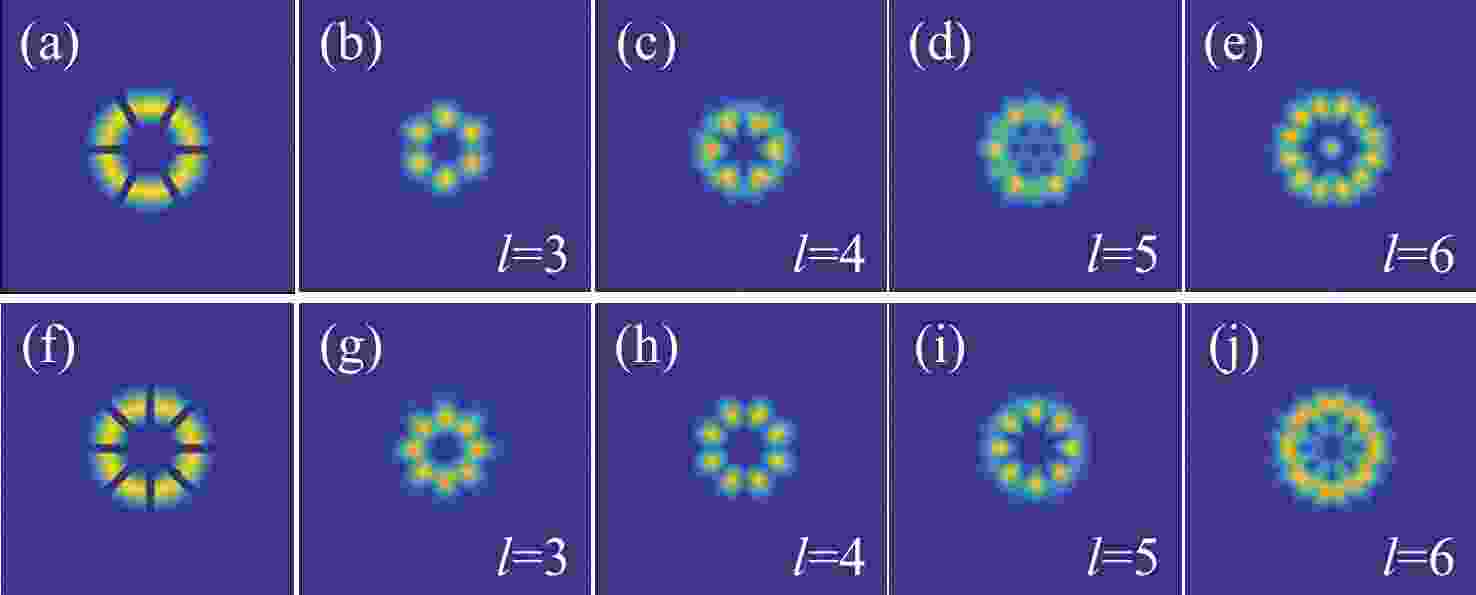
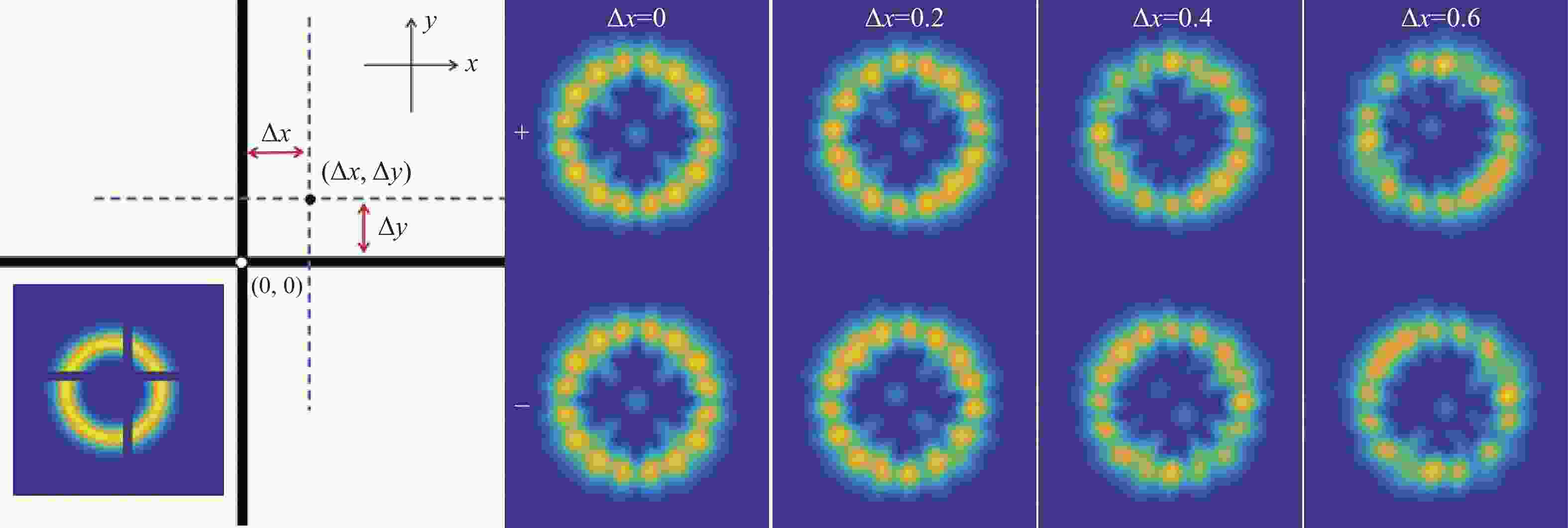
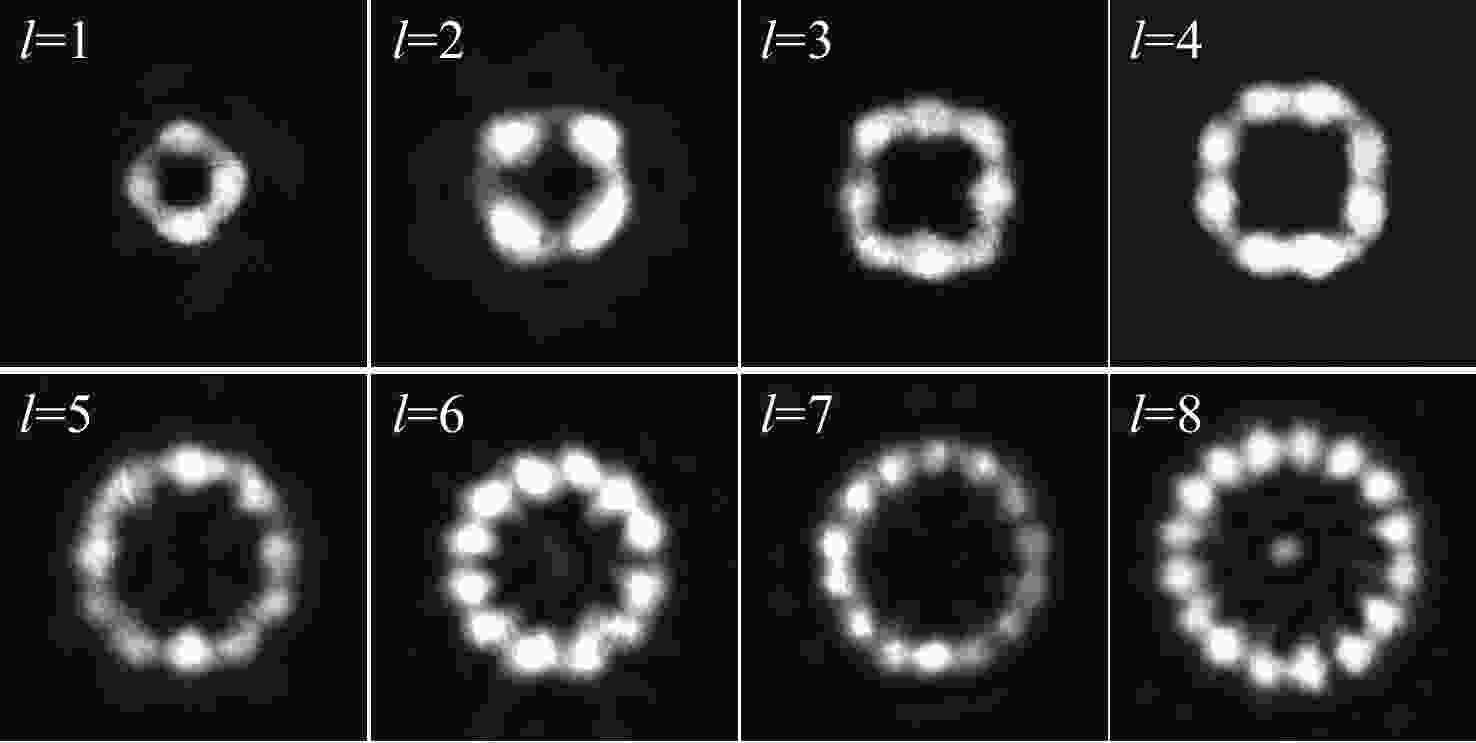
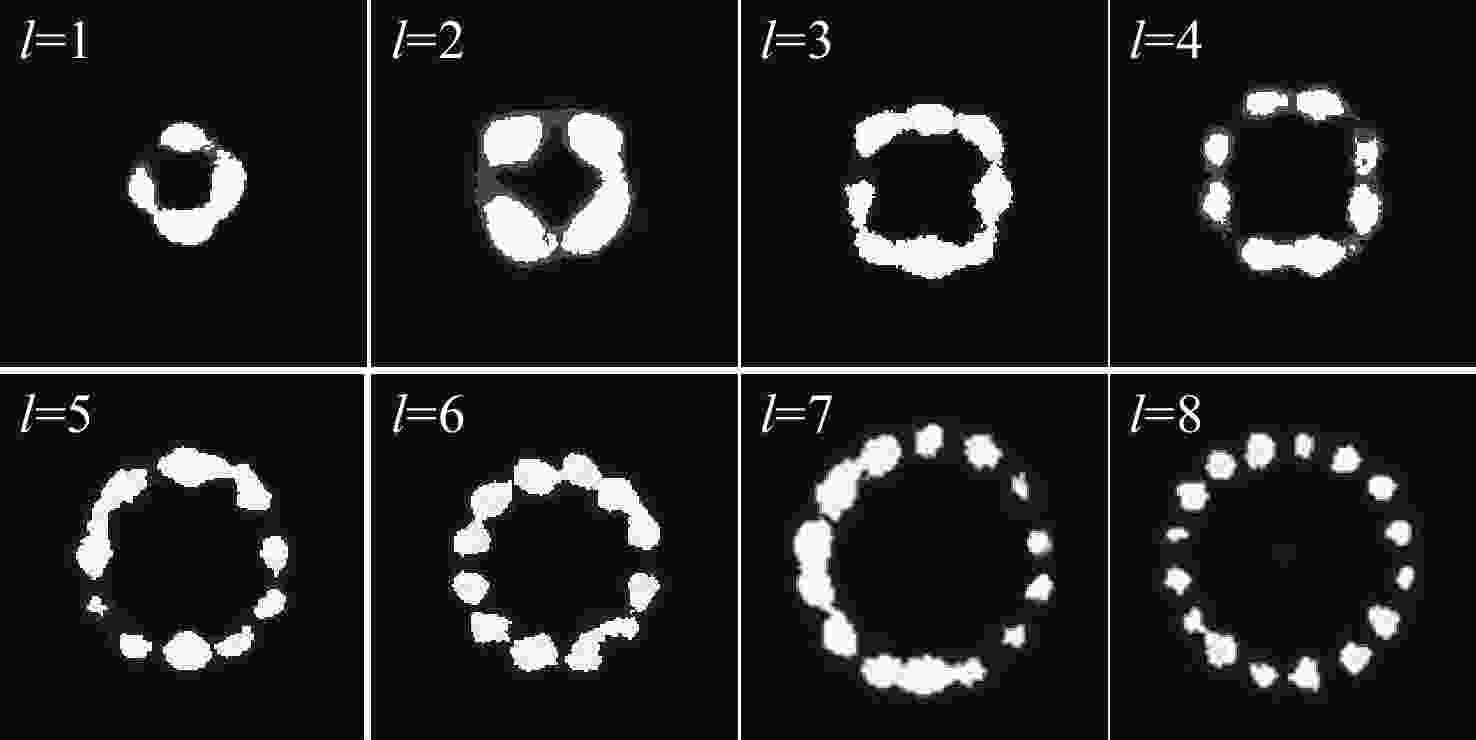
本文提出一种利用十字线衍射进行涡旋光束OAM检测的方法，其远场分布中与OAM相关的主亮斑包含了入射光束的大部分能量（50%~84%），且不存在干扰检测的次亮斑。相比之下，传统小孔衍射法中的主亮斑能量比例极低，尤其是7阶拓扑荷以上的远场主亮斑中只包含不到1%的入射光束能量，且拓扑荷级数越高，次亮斑的干扰性越强。因此，十字线测量法对弱涡旋光束的检测尤为适用，这对于远程的自由空间光通讯发展具有潜在的重要影响。
Abstract:In this paper, a method for vortex beam OAM detection using crosshair diffraction is proposed. The OAM-related main bright spot in the far-field distribution contains most of the energy of the incident beam (50%~84%) and there is no secondary bright spot that interferes with the detection. In contrast, the energy proportion of the main bright spot in the conventional small-hole diffraction method is extremely low, particularly in the far-field main bright spot above the 7th-order topological charge, which contains less than 1% of the energy of the incident beam. Furthermore, as the topological charge level increases, the secondary bright spot becomes more intrusive. Consequently, crosshair measurements are particularly applicable to the detection of weak vortex beams, which has potentially important implications for the development of long-range free-space optical communications.
-
Key words:
- diffraction /
- vortex beam /
- orbital angular momentum /
- topological charge
-
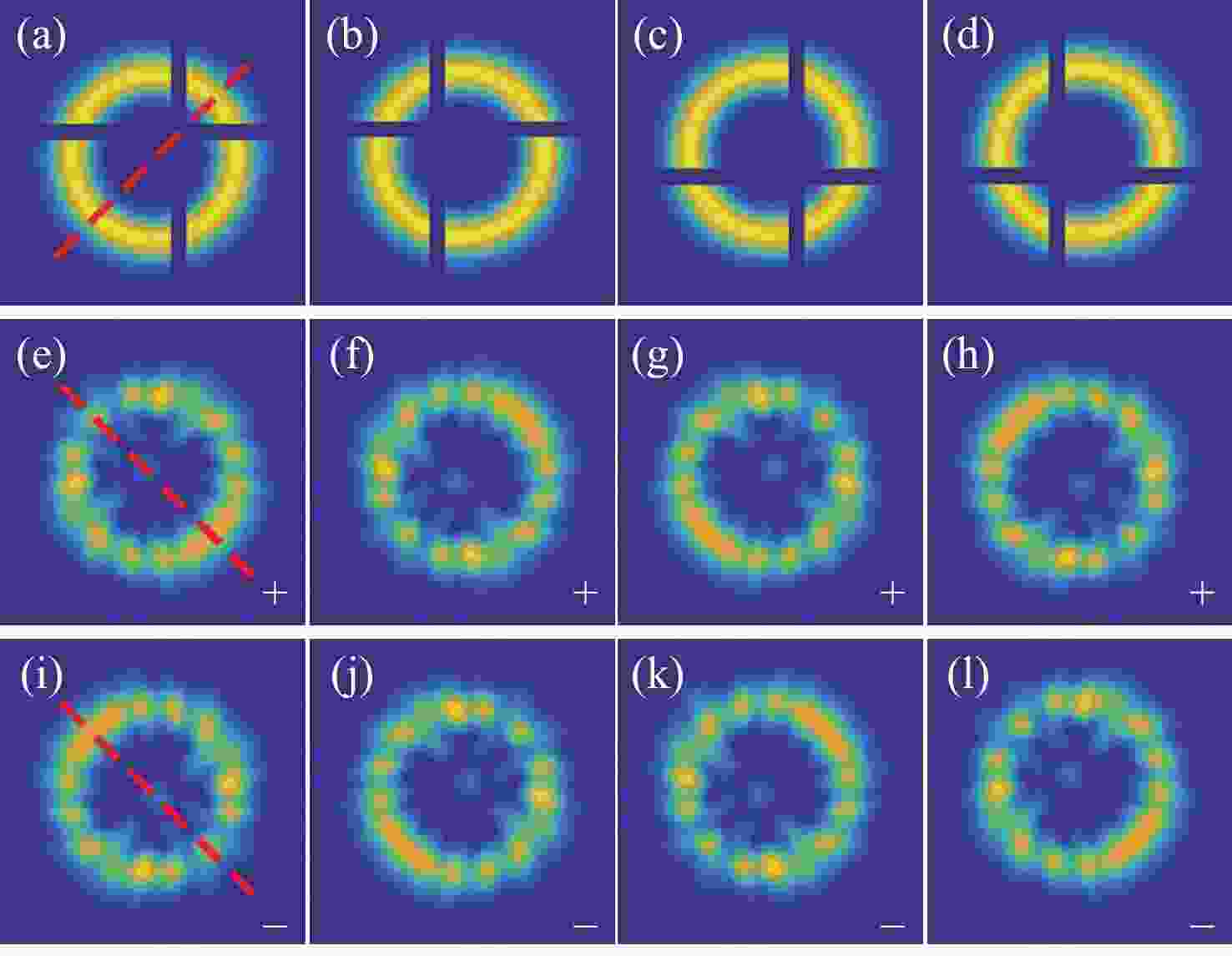
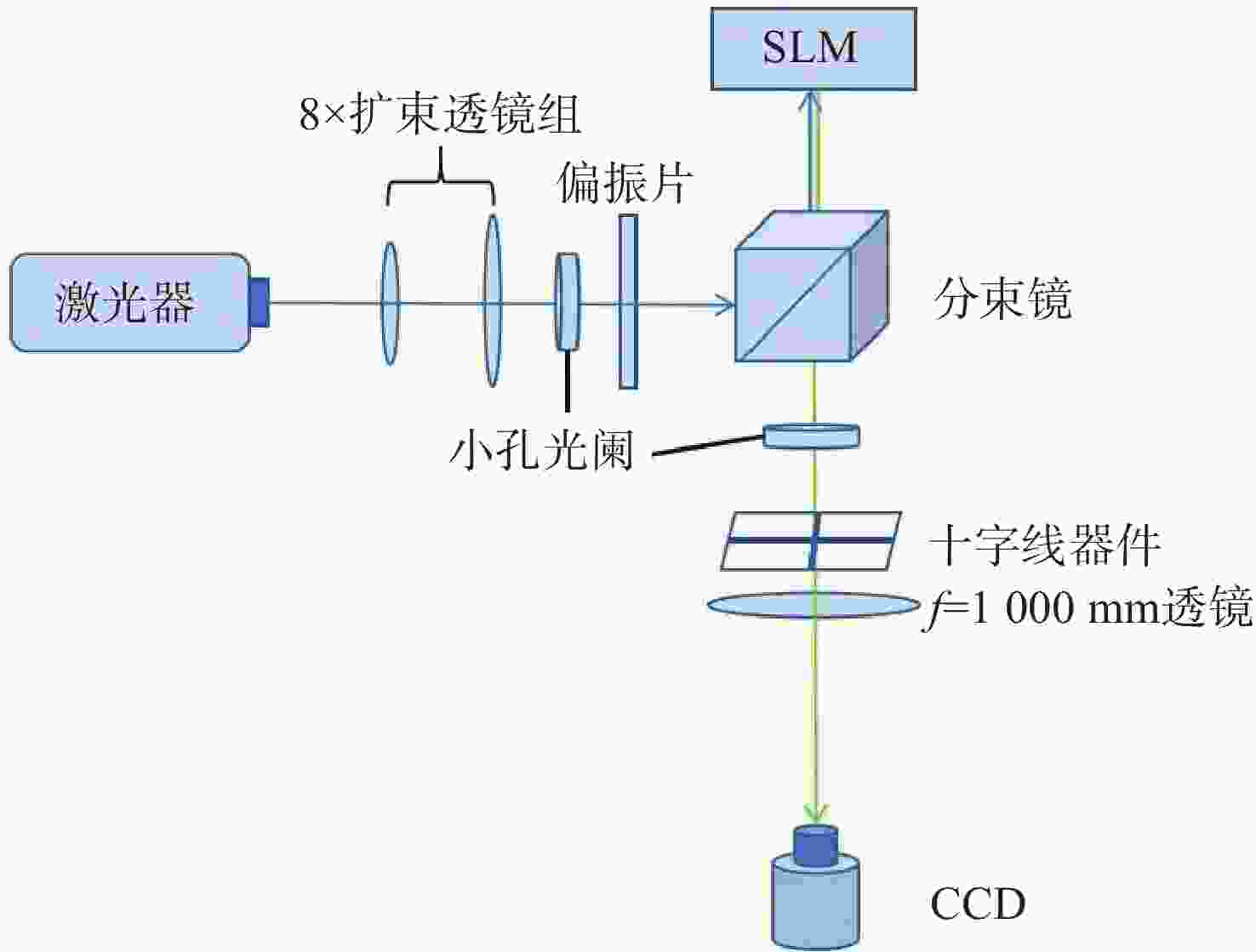
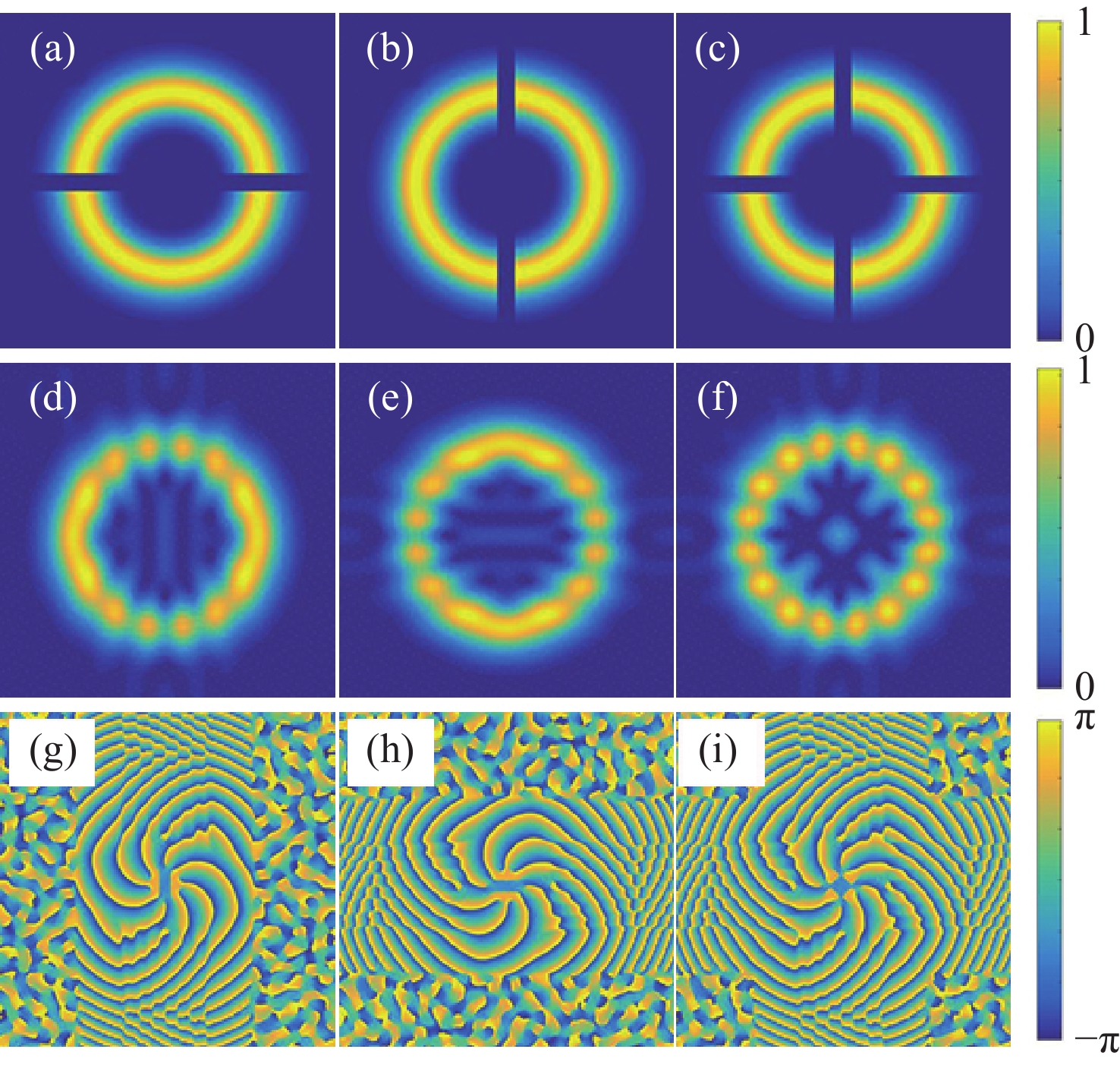
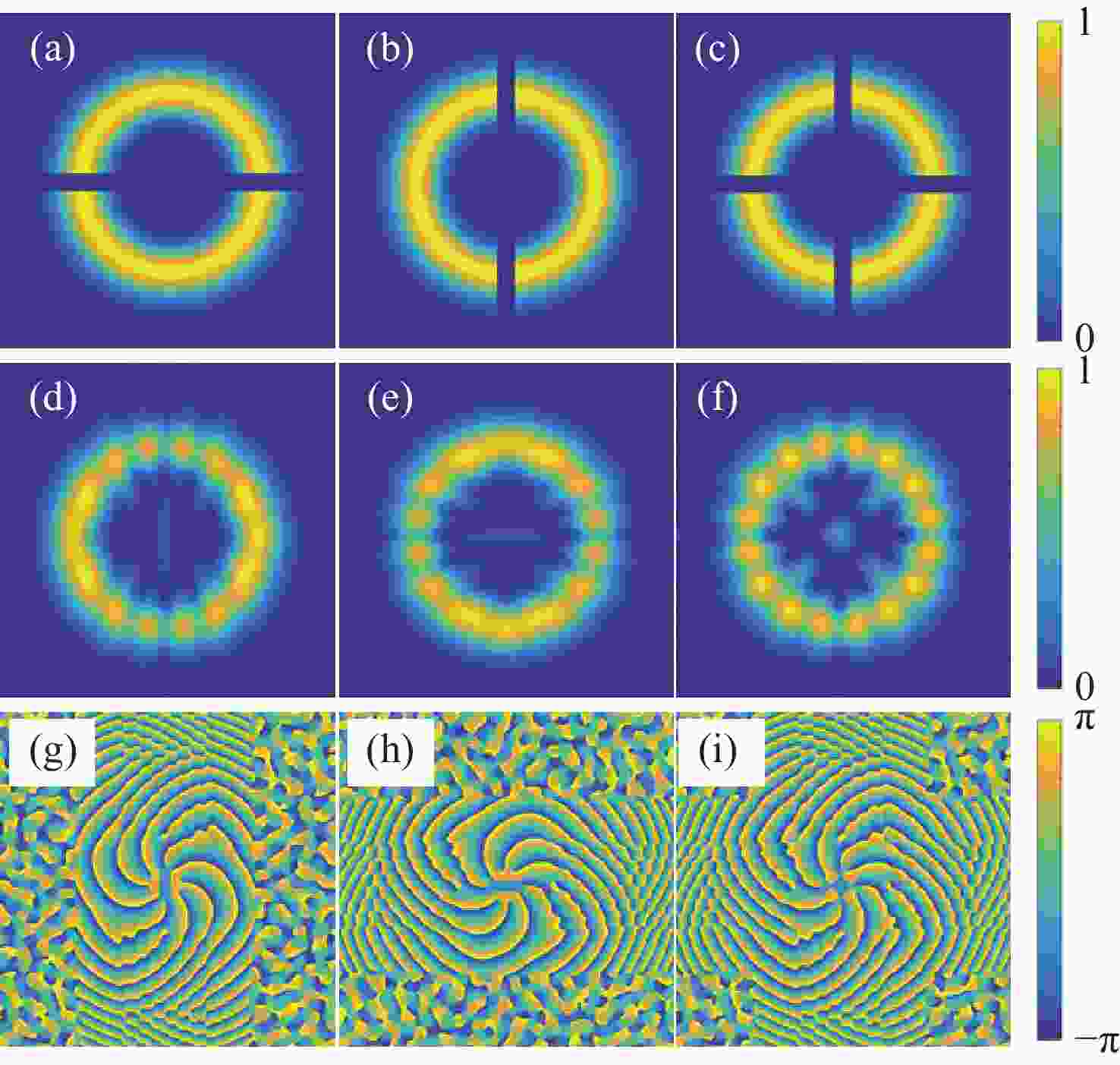
图 1 高斯涡旋光束经不同线结构衍射的远场分布（a）横线；（b）竖线；（c）十字线；（d）~（f）为（a）~（c）线结构对应的远场衍射结果；（g）~（i）为（d）~（f）远场衍射结果的相位分布
Figure 1. Far-field distribution of Gaussian vortex beams diffracted by different line structures. (a) horizontal line; (b) vertical line; (c) crosshair; (d)-(f) are the far-field diffraction results corresponding to (a)-(c) line structures; (g)-(i) is the phase distribution of the far-field diffraction results from (d)-(f)
图 2 十字线检测法的远场衍射结果
Figure 2. Far-field diffraction results of the crosshair detection method
图 3 三条线和米字线结构的远场衍射结果
Figure 3. Far-field diffraction results of three lines and star patterned line structures
图 4 十字线中心位置偏移时的远场衍射结果
Figure 4. Far-field diffraction results when there is an offset in the center position of the crosshair
图 5 改变十字线偏移方向的远场衍射结果
Figure 5. Far-field diffraction results for changing the direction of the crosshair offset
图 6 10阶拓扑荷涡旋光束经不同光阑后的远场衍射分布
Figure 6. Far-field diffraction distribution of a 10th-order topological charge vortex beam through different apertures
图 7 十字线检测法的实验光路示意图
Figure 7. Experimental light path diagram of the crosshair detection method
图 11 十字线偏移情况的实验结果
Figure 11. Experimental results of the crosshair center position offset
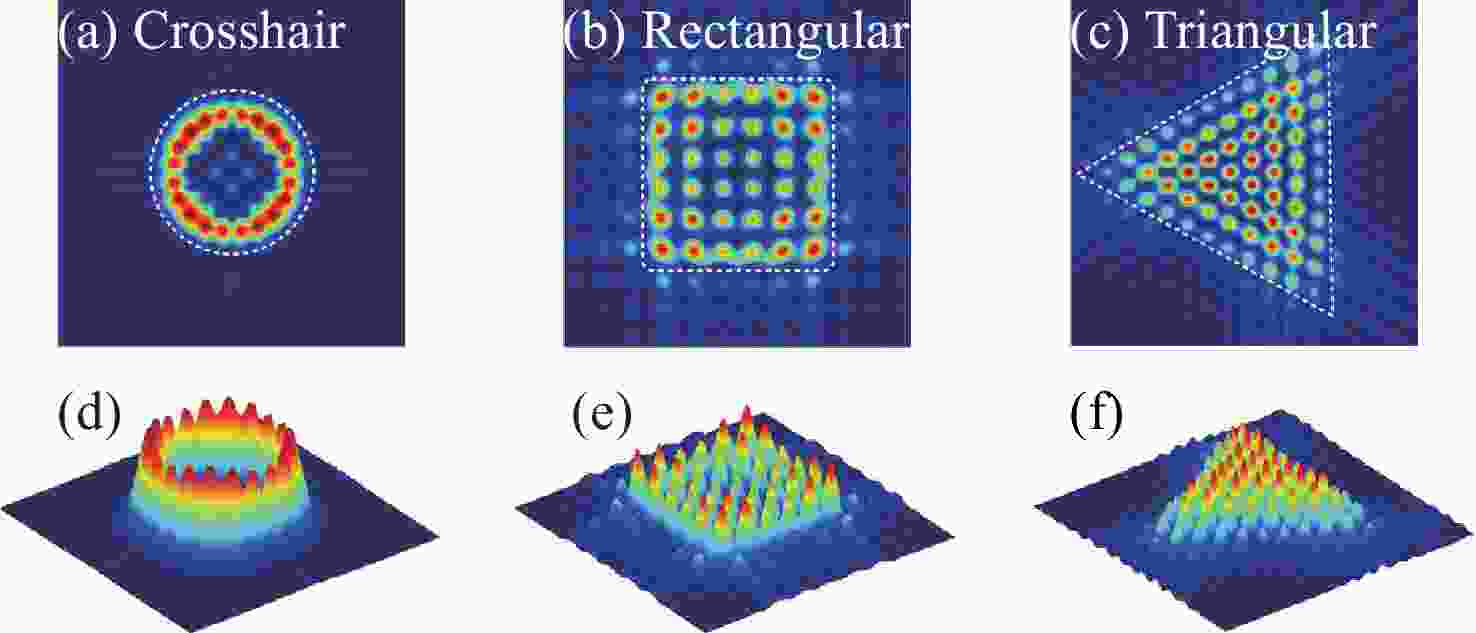
表 1 高斯涡旋光束经不同光阑衍射后的远场主亮斑能量
Table 1. Far-field primary spot energy of Gaussian vortex beams through different apertures
Topological
chargeCross line Rectangular
apertureTriangular
aperture1 50.65% 89.84% 78.05% 2 63.67% 50.27% 44.63% 3 68.63% 21.58% 23.12% 4 74.07% 9.32% 10.84% 5 77.11% 3.81% 5.34% 6 79.37% 1.46% 2.67% 7 81.13% 0.48% 1.25% 8 82.60% 0.16% 0.57% 9 83.77% 0.05% 0.24% 10 84.49% 0.01% 0.10% 
下载: 导出CSV
-
[1] ALLEN L, BEIJERSBERGEN M W, SPREEUW R J C, et al. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes[J]. Physical Review A, 1992, 45(11): 8185-8189. doi: 10.1103/PhysRevA.45.8185 [2] 娄岩, 陈纯毅, 赵义武, 等. 高斯涡旋光束在大气湍流传输中的特性研究[J]. 中国光学,2017,10(6):768-776. doi: 10.3788/co.20171006.0768LOU Y, CHEN CH Y, ZHAO Y W, et al. Characteristics of Gaussian vortex beam in atmospheric turbulence transmission[J]. Chinese Optics, 2017, 10(6): 768-776. (in Chinese). doi: 10.3788/co.20171006.0768 [3] LIU J, CHEN SH, WANG H Y, et al. Amplifying orbital angular momentum modes in ring-core erbium-doped fiber[J]. Research, 2020, 2020: 7623751. [4] LIU J Y, ZHANG J X, LIU J, et al. 1-Pbps orbital angular momentum fibre-optic transmission[J]. Light: Science & Applications, 2022, 11(1): 202. [5] LIU Z Q, ZHANG H K, LIU K G, et al. Data transmission under high scattering based on OAM-basis transmission matrix[J]. Optics Letters, 2022, 47(17): 4580-4583. doi: 10.1364/OL.469688 [6] KONG A R, LEI T, WANG D W, et al. Extending orbital angular momentum multiplexing to radially high orders for massive mode channels in fiber transmission[J]. Optics Letters, 2023, 48(14): 3717-3720. doi: 10.1364/OL.495704 [7] LAMPERSKA W, MASAJADA J, DROBCZYŃSKI S, et al. Optical vortex torque measured with optically trapped microbarbells[J]. Applied Optics, 2020, 59(15): 4703-4707. doi: 10.1364/AO.385167 [8] STUHLMÜLLER N C X, FISCHER T M, DE LAS HERAS D. Colloidal transport in twisted lattices of optical tweezers[J]. Physical Review E, 2022, 106(3-1): 034601. [9] ZENG K, PU J J, XU X M, et al. Gradient torque and its effect on rotational dynamics of optically trapped non-spherical particles in the elliptic Gaussian beam[J]. Optics Express, 2023, 31(10): 16582-16592. doi: 10.1364/OE.488217 [10] WANG J Y, LI F F, KANG G G. Multiwavelength achromatic super-resolution focusing via a metasurface-empowered controlled generation of focused cylindrically polarized vortex beams[J]. Optics Express, 2022, 30(17): 30811-30821. doi: 10.1364/OE.462900 [11] 赵冬娥, 李诺伦, 马亚云, 等. 基于剪切干涉的双涡旋光干涉图样特性研究[J]. 激光与光电子学进展,2021,58(11):1108001.ZHAO D E, LI N L, MA Y Y, et al. Characteristics of double-vortex optical interferogram based on shearing interference[J]. Laser & Optoelectronics Progress, 2021, 58(11): 1108001. (in Chinese). [12] 刘泽隆, 李茂月, 卢新元, 等. 高动态范围条纹结构光在机检测技术及应用进展[J]. 中国光学(中英文),2024,17(1):1-18. doi: 10.37188/CO.2023-0068LIU Z L, LI M Y, LU X Y, et al. On-machine detection technology and application progress of high dynamic range fringe structured light[J]. Chinese Optics, 2024, 17(1): 1-18. (in Chinese). doi: 10.37188/CO.2023-0068 [13] MA J B, LI P, ZHOU Z H, et al. Characteristics of fork-shaped fringes formed by off-axis interference of two vortex beams[J]. Journal of the Optical Society of America A, 2021, 38(1): 115-123. doi: 10.1364/JOSAA.412404 [14] COX M A, CELIK T, GENGA Y, et al. Interferometric orbital angular momentum mode detection in turbulence with deep learning[J]. Applied Optics, 2022, 61(7): D1-D6. doi: 10.1364/AO.444954 [15] QIN H, FU Q, TAN W, et al. Highly accurate OAM mode detection network for ring Airy Gaussian vortex beams disturbed by atmospheric turbulence based on interferometry[J]. Journal of the Optical Society of America A, 2023, 40(7): 1319-1326. doi: 10.1364/JOSAA.491846 [16] SILVA J G, JESUS-SILVA A J, ALENCAR M A R C, et al. Unveiling Square and triangular optical lattices: a comparative study[J]. Optics Letters, 2014, 39(4): 949-952. doi: 10.1364/OL.39.000949 [17] LAN B, LIU CH, RUI D M, et al. The topological charge measurement of the vortex beam based on dislocation self-reference interferometry[J]. Physica Scripta, 2019, 94(5): 055502. doi: 10.1088/1402-4896/ab03a2 [18] CHEN T CH, LU X Y, ZENG J, et al. Young's double-slit experiment with a partially coherent vortex beam[J]. Optics Express, 2020, 28(25): 38106-38114. doi: 10.1364/OE.410812 [19] MELO L A, JESUS-SILVA A J, CHÁVEZ-CERDA S, et al. Direct measurement of the topological charge in elliptical beams using diffraction by a triangular aperture[J]. Scientific Reports, 2018, 8(1): 6370. doi: 10.1038/s41598-018-24928-5 [20] 庄京秋, 熊晗, 虞天成, 等. 基于软边小孔的涡旋光轨道角动量检测研究[J]. 光学 精密工程,2022,30(12):1394-1405. doi: 10.37188/OPE.20223012.1394ZHUANG J Q, XIONG H, YU T CH, et al. Orbital angular momentum detection of vortex beam based on soft-edge aperture[J]. Optics and Precision Engineering, 2022, 30(12): 1394-1405. (in Chinese). doi: 10.37188/OPE.20223012.1394 [21] WANG J Q, FU SH Y, SHANG Z J, et al. Adjusted EfficientNet for the diagnostic of orbital angular momentum spectrum[J]. Optics Letters, 2022, 47(6): 1419-1422. doi: 10.1364/OL.443726 [22] LI X J, SUN L M, HUANG J M, et al. Research on orbital angular momentum recognition technology based on a convolutional neural network[J]. Sensors, 2023, 23(2): 971. doi: 10.3390/s23020971 [23] 谌娟, 柯熙政, 杨一明. 拉盖尔高斯光的衍射和轨道角动量的弥散[J]. 光学学报,2014,34(4):0427001. doi: 10.3788/AOS201434.0427001CHEN J, KE X ZH, YANG Y M. Laguerre-Gaussian beam diffraction and dispersion of the orbital angular momentum[J]. Acta Optica Sinica, 2014, 34(4): 0427001. (in Chinese). doi: 10.3788/AOS201434.0427001 -
下载:
计量
- 文章访问数: 84
- HTML全文浏览量: 18
- PDF下载量: 22
- 被引次数: 0
