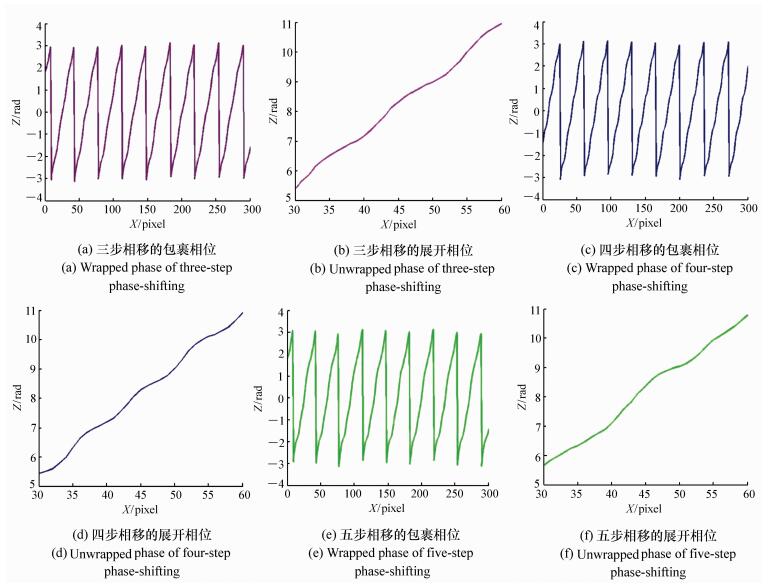
-
摘要: 双N步相移轮廓术虽然可以大大降低由于光栅条纹的非正弦性所导致的相位误差,但增加了一倍的投影条纹数量,降低了测量效率。针对此问题,本文提出一种基于彩色编码光栅投影的双N步相移轮廓术,它将原相移条纹和附加相移条纹编码成双色条纹,融合到一幅彩色光栅条纹中投影,然后从采集的彩色条纹中提取两套条纹的相位信息,分别解包裹相位后,融合两包裹相位以减小相位误差。为验证所提方法的有效性,将该方法与两种典型的相位展开算法结合进行实验。实验结果证明,所提方法能有效降低相位误差,且不需要增加任何额外的光栅条纹,测量效率提高了46%。Abstract: Although double N-step phase-shifting profilometry can greatly reduce phase error caused by the non-sinusoidal nature of grating fringes, its number of the projection fringes doubles and its measurement efficiency is reduced. In this paper, a double N-step phase-shifting profilometry using color-encoded grating projection is proposed. It encodes the original phase-shifting fringes and the additional phase-shifting fringes into two colored fringes and fuses them into one color-encoded grating fringe projection. Then, the phase information of two sets of fringes is extracted from the captured color-encoded fringes. After calculating their wrapped phases, the two wrapped phases are fused to reduce phase error. In order to verify the effectivity of the proposed method, we combine the proposed method with two typical phase unwrapping algorithms to carry out experiments. The experimental results show that the proposed method can effectively reduce the phase error without adding any additional grating fringes and that its measurement efficiency is enhanced by 46%.
-
表 1 条纹数量
Table 1. The number of fringes
算法 双N步相移与枝切法解相位 双N步相移与三频外差法解相位 条纹颜色 灰度条纹 彩色条纹 灰度条纹 彩色条纹 条纹数量 8 4 24 12 -
[1] 张来刚, 魏仲慧, 何昕, 等.多约束融合算法在多摄像机测量系统中的应用[J].液晶与显示, 2013, 28(4):608- 614. http://d.old.wanfangdata.com.cn/Periodical/yjyxs201304025ZHANG L G, WEI ZH H, HE X, et al.. Multi-camera measurement system based on multi-constraint fusion algorithm[J]. Chinese Journal of Liquid Crystals and Displays, 2013, 28(4):608-614.(in China) http://d.old.wanfangdata.com.cn/Periodical/yjyxs201304025 [2] ZHONG K, LI ZH W, SHI Y SH, et al.. Fast phase measurement profilometry for arbitrary shape objects without phase unwrapping[J]. Optics and Lasers in Engineering, 2013, 51(11):1213-1222. doi: 10.1016/j.optlaseng.2013.04.016 [3] 王勇, 饶勤菲, 唐靖, 等.采用小波脊系数幅值导数方差质量图的相位展开法[J].光子学报, 2015, 44(2):0210001. http://d.old.wanfangdata.com.cn/Periodical/gzxb201502011WANG Y, RAO Q F, TANG J, et al.. Phase unwrapping algorithm based on the amplitude of wavelet ridge coefficient variance derivative quality map[J]. Acta Photonica Sinica, 2015, 44(2):0210001.(in Chinese) http://d.old.wanfangdata.com.cn/Periodical/gzxb201502011 [4] 王建华, 杨延西, 马晨.基于价值函数的二维小波变换小波脊提取算法[J].仪器仪表学报, 2017, 38(12):2915-2923. http://www.cnki.com.cn/Article/CJFDTOTAL-YQXB201712005.htmWANG J H, YANG Y X, MA CH. Wavelet ridge extraction algorithm using a cost function in two-dimensional wavelet transform[J]. Chinese Journal of Scientific Instrument, 2017, 38(12):2915-2923.(in Chinese) http://www.cnki.com.cn/Article/CJFDTOTAL-YQXB201712005.htm [5] ZHANG S, YAU S T. High-resolution, real-time 3D absolute coordinate measurement based on a phase-shifting method[J]. Optics Express, 2006, 14(7):2644-2649. doi: 10.1364/OE.14.002644 [6] GAI SH Y, DA F P. A novel phase-shifting method based on strip marker[J]. Optics and Laser in Engineering, 2010, 48(2):205-211. doi: 10.1016/j.optlaseng.2009.03.020 [7] HUANG P S, ZHANG S. Fast three-step phase-shifting algorithm[J]. Applied Optics, 2006, 45(21):5086-5091. doi: 10.1364/AO.45.005086 [8] TAKEDA M, MUTOH K. Fourier transform profilometry for the automatic measurement of 3-D object shapes[J]. Applied Optics, 1983, 22(24):3977-3982. doi: 10.1364/AO.22.003977 [9] ABID A. Fringe pattern demodulation using the one-dimensional continuous wavelet transform: field-programmable gate array implementation[J]. Applied Optics, 2013, 52(7):1468-1471. doi: 10.1364/AO.52.001468 [10] WANG ZH Y, MA J, VO M. Recent progress in two-dimensional continuous wavelet transform technique for fringe pattern analysis[J]. Optics and Lasers in Engineering, 2012, 50(8):1052-1058. doi: 10.1016/j.optlaseng.2012.01.029 [11] ZHANG Z B, ZHONG J G. Applicability analysis of wavelet-transform profilometry[J]. Optics Express, 2013, 21(16):18777-18796. doi: 10.1364/OE.21.018777 [12] ZHANG S, YAU S T. Generic nonsinusoidal phase error correction for three-dimensional shape measurement using a digital video projector[J]. Applied Optics, 2007, 46(1):36-43. doi: 10.1364/AO.46.000036 [13] BAKER M J, XI J, CHICHARO J F. Elimination of gamma nonlinear luminance effects for digital video projection phase measuring profilometers[C]. Proceedings of the 4th IEEE International Symposium on Electronic Design, Test and Applications, 2008: 496-501. [14] PAN B, QIAN K M, HUANG L, et al.. Phase error analysis and compensation for nonsinusoidal waveforms in phase-shifting digital fringe projection profilometry[J]. Optics Letter, 2009, 34(4):416-418. doi: 10.1364/OL.34.000416 [15] CAI Z W, LIU X L, LIANG H, et al.. Flexible phase error compensation based on Hilbert transform in phase shifting profilometry[J]. Optics Express, 2015, 23(19):25171-25181. doi: 10.1364/OE.23.025171 [16] YATABE K, ISHIKAWA K, OIKAWA Y. Compensation of fringe distortion for phase-shifting three-dimensional shape measurement by inverse map estimation[J]. Applied Optics, 2016, 55(22):6017-6024. doi: 10.1364/AO.55.006017 [17] ZHANG W, YU L D, LI W SH, et al.. Black-box phase error compensation for digital phase-shifting profilometry[J]. IEEE Transactions on Instrumentation and Measurement, 2017, 66(10):2755-2761. doi: 10.1109/TIM.2017.2712862 [18] HUANG P S, HU Q J, CHIANG F P. Double three-step phase-shifting algorithm[J]. Applied Optics, 2002, 41(22):4503-4509. doi: 10.1364/AO.41.004503 [19] SU W H. Color-encoded fringe projection for 3D shape measurements[J]. Optics Express, 2007, 15(20):13167-13181. doi: 10.1364/OE.15.013167 [20] JIANG CH, JIA SH H, DONG J, et al.. Multi-frequency color-marked fringe projection profilometry for fast 3D shape measurement of complex objects[J]. Optics Express, 2015, 23(19):24152-24162. doi: 10.1364/OE.23.024152 [21] SONG L M, LI Z Y, CHEN CH M, et al. A correction method of color projection fringes in 3D contour measurement[J]. Optoelectronics Letters, 2015, 11(4):303-306. doi: 10.1007/s11801-015-5050-x [22] GOLDSTEIN R M, ZEBKER A, WERNER C A, et al.. Satelliteradar interferometry:two dimensional phase unwrapping[J]. Radio Science, 1988, 23(4):713-720. doi: 10.1029/RS023i004p00713 [23] ZUO CH, HUANG L, ZHANG M L, et al.. Temporal phase unwrapping algorithms for fringe projection profilometry:a comparative review[J]. Optics and Lasers in Engineering, 2016, 85:84-103. doi: 10.1016/j.optlaseng.2016.04.022 [24] NGUYEN H, NGUYEN D, WANG ZH Y, et al.. Real-time, high-accuracy 3D imaging and shape measurement[J]. Applied Optics, 2015, 54(1):A9-A17. doi: 10.1364/AO.54.0000A9 [25] 孙辉, 柏旭光, 孙丽娜, 等.Bayer图像色彩还原线性插值方法[J].液晶与显示, 2013, 28(3):417-423. http://d.old.wanfangdata.com.cn/Periodical/yjyxs201303022SUN H, BAI X G, SUN L N, et al.. Linear interpolation of demosaicing for bayer pattern[J]. Chinese Journal of Liquid Crystals and Displays, 2013, 28(3):417-423.(in Chinese) http://d.old.wanfangdata.com.cn/Periodical/yjyxs201303022 [26] ZHANG ZH, TOWERS C E, TOWERS D P. Shape and color measurement of colorful objects by fringe projection[J]. Proceedings of SPIE, 2008, 7063:70630N. doi: 10.1117/12.794561 -






 下载:
下载: