空间引力波探测任务的入轨误差分析

李卓; 王有亮; 郑建华; 李明涛

doi:10.3788/CO.20191203.0493

空间引力波探测任务的入轨误差分析

doi: 10.3788/CO.20191203.0493

李卓^{1, 2,},
王有亮¹,
郑建华^{1, 2},
李明涛^{1, 2, ,}

1.
中国科学院国家空间科学中心, 北京 100190
2.
中国科学院大学, 北京 100039

基金项目:

中国科学院战略性先导科技专项 XDA15014901

详细信息

作者简介:
李卓(1992-), 女, 黑龙江大庆人, 博士研究生, 主要从事航天器轨道设计与优化方面的研究。E-mail:15046084839@163.com

李明涛(1982—)，男，河南商丘人，博士，研究员，博士生导师，主要从事航天器轨道动力学与控制方面的研究。E-mail: limingtao@nssc.ac.cn

中图分类号: V11
计量
- 文章访问数: 2710
- HTML全文浏览量: 848
- PDF下载量: 177
- 被引次数: 0
出版历程
- 收稿日期: 2019-02-01
- 修回日期: 2019-04-01
- 刊出日期: 2019-06-01

Injection error analysis of space gravitational wave detection

LI Zhuo^{1, 2
,},
WANG You-liang¹,
ZHENG Jian-hua^{1, 2},
LI Ming-tao^{1, 2
, ,}

1.
National Space Science Center, Chinese Academy of Sciences, Beijing 100190, China
2.
University of Chinese Academy of Sciences, Beijing 100039, China

Funds:

the Strategy Priority Research Program of the Chinese Academy of Sciences XDA15014901

More Information

Corresponding author: LI Ming-tao, limingtao@nssc.ac.cn

摘要

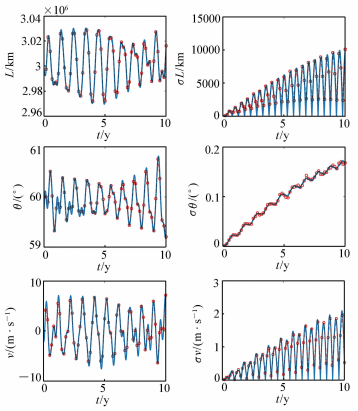
摘要: 空间引力波探测任务要求构型在长达几年的工作过程中保持高水平稳定，而入轨误差可能会破坏构型的稳定性。因此本文研究了入轨误差对构型的影响。首先，分别用Monte-Carlo法和协方差分析函数描述法（Covariance Analysis Description Equation Technique，CADET）进行对照实验，验证了CADET法的正确性。运用CADET法分别研究了位置速度误差大小和方向对构型的影响。实验结果表明：CADET法具有计算效率高的优点，并且相对误差不超过6%；径向位置误差和切向速度误差对构型影响显著；当编队卫星位置和速度误差相对方向相同时对构型的影响更小；两种误差同时存在时，当位置误差不超过160 km，速度误差不超过3 cm/s时构型可维持稳定。CADET法准确有效且高速，适用于空间引力波探测任务的入轨误差分析。
- 空间引力波探测任务 /
- 入轨误差 /
- 构型稳定性 /
- CADET法
Abstract: In order to analyze the effect of injection error on gravitational wave detection, and keep arm length, breathing angle, arm length variation rate and distance to earth acceptable, the effect of injection error is investigated. First, the Monte-Carlo and CADET are tested and compared. CADET is proved to be correct. The effects of position and velocity error on constellations are researched with the CADET method. Experimental results indicate that the relative error between CADET and Monte-Carlo is less than 6%, and the calculated time of CADET is less than 1 min. Radial position error and tangential velocity error have a greater effect on constellation. If the position error of the three satellites are in same direction, maintaining stability is easier. The same is true for velocity error. A constellation can remain stable when position error is no more than 160 km and velocity error is no more than 3 cm/s. CADET is appropriate for injection error analysis because of its accuracy and high efficiency.
- gravitational wave observatory /
- injection error /
- constellation stability /
- CADET

HTML全文

图 1 CADET法和Monte-Carlo法的对照结果

Figure 1. Comparison diagrams of CADET and Monte-Carlo

下载: 全尺寸图片幻灯片

图 2 初始构型

Figure 2. Initial constellations

下载: 全尺寸图片幻灯片

图 3 位置误差与速度误差的关系

Figure 3. Relationship between position error and velocity error

下载: 全尺寸图片幻灯片

表 1 CADET法和Monte-Carlo法结果比较

Table 1. Results comparison of CADET and Monte-Carlo methods

		L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
均值	MC	3 016 317.792 1	3 021 446.315 6	2 982 485.042 0	59.204 0	60.483 7	60.312 1	7.168 2	-3.707 0	4.349 5
	CADET	3 015 780.761 7	3 020 993.339 8	2 981 955.221 9	59.205 8	60.490 7	60.303 4	7.147 0	-3.699 5	4.278 4
标准差	MC	10 089.131 3	7 639.225 8	2 603.269 3	0.168 1	0.182 8	0.176 9	0.525 3	1.460 3	2.142 0
	CADET	9 897.729 5	7 600.800 5	2 457.476 2	0.168 8	0.174 0	0.170 2	0.529 8	1.444 2	2.027 6

下载: 导出CSV

表 2 位置误差方向对构型的影响

Table 2. Effect of position error direction on constellation

		L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
径向	均值	3 014 428.590 4	3 014 411.924 5	3 014 041.631 1	60.328 4	60.365 1	60.369 2	3.334 9	3.260 2	3.307 2
	标准差	3 117.635 1	3 413.799 0	3 306.621 0	0.055 8	0.056 6	0.055 9	0.653 4	0.635 7	0.670 9
切向	均值	3 014 324.06 47	3 014 310.481 6	3 014 323.441 0	60.333 3	60.364 9	60.367 4	3.321 5	3.254 7	3.307 3
	标准差	91.243 0	91.886 3	72.738 5	0.001 5	0.001 5	0.001 5	0.021 6	0.021 5	0.019 6
法向	均值	3 014 354.154 6	3 014 250.519 3	3 014 323.744 1	60.332 7	60.364 6	60.367 8	3.325 2	3.252 1	3.307 3
	标准差	30.993 0	30.414 5	40.810 0	0.000 6	0.000 6	0.000 6	0.015 0	0.015 2	0.008 2

下载: 导出CSV

表 3 速度误差方向对构型的影响

Table 3. Effect of velocity error direction on constellation

		L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
径向	均值	3 014 364.693 7	3 014 218.896 0	3 014 324.092 7	60.332 4	60.364 5	60.368 0	3.326 2	3.250 8	3.307 2
	标准差	90.319 9	90.119 2	57.271 8	0.001 5	0.001 5	0.001 5	0.016 8	0.016 7	0.018 7
切向	均值	3 014 358.196 1	3 014 265.270 6	3 014 292.517 4	60.332 2	60.364 7	60.367 9	3.326 1	3.253 3	3.307 2
	标准差	1 589.610 0	1 728.196 7	1 696.484 1	0.028 2	0.028 6	0.028 5	0.341 0	0.331 4	0.337 9
法向	均值	3 014 351.696 3	3 014 250.564 6	3 014 322.426 1	60.332 7	60.364 7	60.367 8	3.325 1	3.252 8	3.307 2
	标准差	19.192 6	19.225 4	10.546 9	0.000 3	0.000 3	0.000 3	0.006 1	0.006 0	0.008 1

下载: 导出CSV

表 4 误差大小对构型的影响(周期4年)

Table 4. Effect of error magnitude on constellation(T=4 years)

			L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
位置误差 (km)	100	均值	3 014 428.590 4	3 014 411.924 5	3 014 041.631 1	60.328 4	60.365 1	60.369 2	3.334 9	3.260 2	3.307 2
	100	标准差	3 117.635 1	3 413.799 0	3 306.621 0	0.055 8	0.056 6	0.055 9	0.653 4	0.635 7	0.670 9
	1 000	均值	3 015 125.864 9	3 015 879.464 1	3 011 524.225 5	60.290 0	60.395 3	60.382 6	3.426 0	3.329 6	3.307 1
	1 000	标准差	31 181.532 3	34 145.594 2	33 072.501 0	0.558 2	0.566 5	0.558 4	6.536 3	6.357 7	6.709 2
速度误差 (cm/s)	1	均值	3 014 358.196 1	3 014 265.270 6	3 014 292.517 4	60.332 2	60.364 7	60.367 9	3.326 1	3.253 3	3.307 2
	1	标准差	1 589.610 0	1 728.196 7	1 696.484 1	0.028 2	0.028 6	0.028 5	0.341 0	0.331 4	0.337 9
	10	均值	3 014 420.979 4	3 014 400.901 6	3 014 015.281 3	60.327 8	60.365 1	60.368 9	3.337 6	3.261 3	3.307 2
	10	标准差	15 896.848 4	17 283.056 3	16 965.747 2	0.282 5	0.286 6	0.285 2	3.410 3	3.314 5	3.379 6

下载: 导出CSV

表 5 误差大小对构型的影响(周期10年)

Table 5. Effect of error magnitude on constellation(T=10 years)

			L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
位置误差 (km)	100	均值	3 030 326.996 2	3 026 882.999 7	3 029 905.409 1	60.812 6	60.961 1	60.837 5	7.141 1	7.289 3	6.943 0
	100	标准差	6 605.262 9	7 012.686 8	6 858.372 8	0.113 9	0.116 9	0.115 6	1.368 2	1.331 3	1.399 6
	1 000	均值	3 030 641.096 1	3 032 443.748 6	3 032 228.912 6	60.794 5	61.047 3	60.880 9	7.094 2	7.369 7	7.211 6
	1 000	标准差	66 186.719 5	70 279.365 3	68 727.030 2	1.142 7	1.172 6	1.156 9	13.711 8	13.338 2	14.028 1
速度误差 (cm/s)	1	均值	3 030 296.274 1	3 026 334.210 6	3 029 681.672 2	60.814 6	60.960 2	60.833 2	7.153 9	7.281 6	6.943 0
	1	标准差	3 302.646 5	3 514.742 4	3 443.595 4	0.057 4	0.058 3	0.058 0	0.697 2	0.671 0	0.701 9
	10	均值	3 030 333.464 7	3 026 914.277 1	3 029 929.590 2	60.810 2	60.961 1	60.837 9	7.122 8	7.292 2	6.943 0
	10	标准差	33 043.461 5	35 166.530 0	34 453.912 5	0.574 7	0.584 1	0.580 2	6.975 9	6.713 4	7.023 3

下载: 导出CSV

表 6 相对位置误差方向对构型的影响

Table 6. Effect of direction of relative position error on constellation

		L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
同向	均值	3 014 404.364 8	3 014 472.578 8	3 014 042.126 2	60.328 9	60.365 3	60.368 9	3.332 0	3.262 1	3.307 4
	标准差	3 118.707 5	3 413.855 9	3 307.072 1	0.055 8	0.056 6	0.055 9	0.653 6	0.635 9	0.670 9
反向	均值	3 014 880.772 5	3 017 790.780 4	3 036 402.176 6	60.615 7	60.450 6	60.691 8	3.330 4	2.701 4	6.571 9
	标准差	3 118.703 5	3 413.773 0	3 306.951 0	0.056 2	0.056 9	0.056 8	0.653 6	0.636 6	0.671 9

下载: 导出CSV

表 7 相对速度误差方向对构型的影响

Table 7. Effect of direction of relative velocity error on constellation

		L₁₂/km	L₁₃/km	L₂₃/km	θ₁/(°)	θ₂/(°)	θ₃/(°)	v₁₂/ (m·s^-1)	v₁₃/ (m·s^-1)	v₂₃/ (m·s^-1)
同向	均值	3 014 560.508 0	3 014 090.939 2	3 014 013.501 6	60.324 7	60.363 6	60.371 0	3.354 8	3.249 2	3.307 0
	标准差	15 919.053 4	17 283.325 7	16 969.347 4	0.282 5	0.286 7	0.285 3	3.410 6	3.316 8	3.379 7
反向	均值	3 056 876.709 7	3 086 759.715 3	3 016 355.104 0	61.326 9	61.622 6	60.953 2	12.264 3	14.365 1	3.378 8
	标准差	15 914.563 5	17 282.0559	16 969.318 3	0.282 3	0.287 8	0.283 0	3.408 2	3.310 1	3.379 4

下载: 导出CSV

参考文献(12)

[1]	黄双林, 龚雪飞, 徐鹏, 等.空间引力波探测——天文学的一个新窗口[J].中国科学:物理学力学天文学, 2017, 47(1):010404. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgkx-cg201701004 HUANG SH L, GONG X F, XU P, et al.. Gravitational wave detection in space—a new window in astronomy[J]. Scientia Sinica Physica, Mechanica & Astronomica, 2017, 47(1):010404.(in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgkx-cg201701004
[2]	罗子人, 白姗, 边星, 等.空间激光干涉引力波探测[J].力学进展, 2013, 43(4):415-447. http://d.old.wanfangdata.com.cn/Periodical/twxjz201501004 LUO Z R, BAI SH, BIAN X, et al.. Gravitational wave detection by space laser interferometry[J]. Advances in Mechanics, 2013, 43(4):415-447.(in Chinese) http://d.old.wanfangdata.com.cn/Periodical/twxjz201501004
[3]	王智, 马军, 李静秋.空间引力波探测计划-LISA系统设计要点[J].中国光学, 2015, 8(6):980-987. http://www.chineseoptics.net.cn/CN/abstract/abstract9334.shtml WANG ZH, MA J, LI J Q. Space-based gravitational wave detection mission:design highlights of LISA system[J]. Chinese Optics, 2015, 8(6):980-987.(in Chinese) http://www.chineseoptics.net.cn/CN/abstract/abstract9334.shtml
[4]	LUO J, CHEN L SH, DUAN H Z. TianQin:a space-borne gravitational wave detector[J]. Classical and Quantum Gravity, 2016, 33(3):035010. doi: 10.1088/0264-9381/33/3/035010
[5]	HU X CH, LI X H, WANG Y F, et al.. Fundamentals of the orbit and response for TianQin[J]. Classical and Quantum Gravity, 2018, 35(9):095008. doi: 10.1088/1361-6382/aab52f
[6]	LUO Y ZH, YANG ZH. A review of uncertainty propagation in orbital mechanics[J]. Progress in Aerospace Sciences, 2017, 89:23-39. doi: 10.1016/j.paerosci.2016.12.002
[7]	罗绪盛, 荆武兴, 高长生.火星探测捕获的误差传播分析[J].系统工程与电子技术, 2017, 39(7):1590-1595. http://d.old.wanfangdata.com.cn/Periodical/xtgcydzjs201707023 LUO X SH, JING W X, GAO CH SH. Error propagation analysis of capture for Mars exploration[J]. Systems Engineering and Electronics, 2017, 39(7):1590-1595.(in Chinese) http://d.old.wanfangdata.com.cn/Periodical/xtgcydzjs201707023
[8]	夏炎.引力波探测计划LISA的任务轨道设计与优化[D].南京: 中国科学院紫金山天文台, 2009. XIA Y. Orbit design and optimization for the LISA gravitational wave observatory[D]. Nanjing: Purple Mountain Observatory, Chinese Academy of Sciences, 2009.(in Chinese)
[9]	王有亮.卫星编队飞行相对轨迹优化与控制[D].北京: 中国科学院大学, 2018. WANG Y L. Relative trajectory optimization and control for satellite formation flying[D]. Beijing: University of Chinese Academy of Sciences, 2018.(in Chinese)
[10]	陈磊, 韩蕾, 白显宗, 等.空间目标轨道力学与误差分析[M].北京:国防工业出版社, 2010. CHEN L, HAN L, BAI X Z, et al.. Orbital Dynamics and Error Analysis of Space Object[M]. Beijing:National Defense Industry Press, 2010.(in Chinese)
[11]	张兵.大气层外动能拦截器末制导段性能研究[D].长沙: 国防科技大学, 2005. ZHANG B. End-game performance analysis of exo-atmospheric kinetic vehicle[D]. Changsha: National University of Defense Technology, 2005.(in Chinese)
[12]	梁立波, 罗亚中, 杏建军, 等.基于协方差分析描述函数法的非线性交会精度分析[J].系统工程与电子技术, 2010, 32(9):1977-1981. doi: 10.3969/j.issn.1001-506X.2010.09.40 LIANG L B, LUO Y ZH, XING J J, et al.. Precision analysis of nonlinear rendezvous by covariance analysis description equation technique[J]. Systems Engineering and Electronics, 2010, 32(9):1977-1981.(in Chinese). doi: 10.3969/j.issn.1001-506X.2010.09.40