测试质量干涉仪抖动光程耦合噪声的多因素影响分析

赵梦园; 沈嘉; 彭晓东; 马晓珊; 杨震; 刘河山; 孟新; 张佳锋

doi:10.37188/CO.EN-2024-0031

测试质量干涉仪抖动光程耦合噪声的多因素影响分析

赵梦园^{1, 2,},
沈嘉^{3, 4},
彭晓东^{2, 5, 6, 7},
马晓珊^8, ,,
杨震²,
刘河山³,
孟新²,
张佳锋^{2, 4}

1.
西安财经大学信息学院, 陕西西安 710100
2.
中国科学院国家空间科学中心复杂航天系统电子信息技术重点实验室, 北京 100190
3.
中国科学院力学研究所, 北京 100190
4.
中国科学院大学, 北京 100049
5.
引力波宇宙太极实验室, 浙江杭州 310024
6.
浙江省引力波精密测量重点实验室, 浙江杭州 310024
7.
国科大杭州高等研究院基础物理与数学科学学院, 浙江杭州 310024
8.
中国科学院工程热物理研究所, 北京 100190

详细信息

中图分类号: TP394.1;TH691.9
计量
- 文章访问数: 76
- HTML全文浏览量: 23
- PDF下载量: 3
- 被引次数: 0
出版历程
- 收稿日期: 2024-09-26
- 录用日期: 2024-12-10
- 网络出版日期: 2025-01-22

Analysis of tilt-to-length coupling noise: Exploring the influence of multiple factors in test mass interferometers

doi: 10.37188/CO.EN-2024-0031

ZHAO Meng-yuan^{1, 2
,},
SHEN Jia^{3, 4},
PENG Xiao-dong^{2, 5, 6, 7},
MA Xiao-shan^{8
, ,},
YANG Zhen²,
LIU He-shan³,
MENG Xin²,
ZHANG Jia-feng^{2, 4}

1.
School of Information, Xi'an University of Finance and Economics, Xi'an 710100, P.R. China
2.
Key Laboratory of Electronics and Information Technology for Space System, National Space Science Center, Chinese Academy of Sciences, Beijing 100190, P.R. China
3.
Institute of Mechanics, Chinese Academy of Sciences, Beijing 100190, P.R. China
4.
University of Chinese Academy of Sciences, Beijing 100049, P.R. China
5.
Taiji Laboratory for Gravitational Wave Universe, Hangzhou 310024, P.R. China
6.
Key Laboratory of Gravitational Wave Precision Measurement of Zhejiang Province, Hangzhou 310024, P.R. China
7.
School of Fundamental Physics and Mathematical Sciences, Hangzhou Institute for Advanced Study, UCAS, Hangzhou 310024, P.R. China
8.
Institute of Engineering Thermophysics, Chinese Academy of Sciences, Beijing 100190, P.R. China

Funds: Supported by

More Information

Author Bio:
ZHAO Meng-yuan (1996—), a PhD graduate from Key Laboratory of Electronics and Information Technology for Space Systems in the National Space Science Center, Chinese Academy of Sciences, and is currently employed at the School of Information, Xi’an University of Finance and Economics. Her research interest is the high-precision simulation, analysis, and evaluation of space missions. E-mail: 2023010027@xaufe.edu.cn

MA Xiao-shan, a professor at the Institute of Engineering Thermophysics, Chinese Academy of Sciences. Her research interest is combustion diagnostic technology. E-mail: maxiaoshan@iet.cn

Corresponding author: maxiaoshan@iet.cn

摘要

摘要:
在基于外差干涉原理的空间引力波探测任务中，抖动光程耦合噪声是一个重要的光学噪声源，对测量系统的精度产生显著影响。本文提出了一种分析多种因素共同作用下抖动光程耦合噪声的方法。首先设计了一个等效的测试质量干涉仪仿真光学平台，并采用高斯光束追踪模拟光束传播。通过模拟干涉信号，可以分析各种因素对抖动光程耦合噪声的影响，包括位置因素、光束参数因素、探测器参数因素和信号定义因素。在此基础上，在满足分析要求的参数范围内,构建了由多个影响因素组成的随机参数空间，并通过基于方差的全局敏感性分析对随机采样得到的模拟结果进行评估。主要效应指数和总效应指数的计算结果表明，测试质量的旋转角度和活塞效应（径向）对测试质量干涉仪中的抖动光程耦合噪声有显著影响。这一结论为空间激光干涉测量系统的设计和优化提供了定性参考。
- 空间干涉测量 /
- 光学仿真 /
- 抖动光程耦合噪声
Abstract:
For space-borne gravitational wave detection missions based on the heterodyne interferometry principle, tilt-to-length (TTL) coupling noise is an important optical noise source, significantly influencing the accuracy of the measurement system. This paper presents a method for analyzing TTL coupling noise under the joint influence of multiple factors. An equivalent simulated optical bench for the test mass interferometer was designed, and Gaussian beam tracing was adopted to simulate beam propagation. By simulating the interference signal, it can analyze the impact of various factors on the TTL coupling noise, including positional, beam parameters, detector parameters, and signal definition factors. On this basis, a random parameter space composed of multiple influential factors was constructed within a range satisfying the analysis requirement, and the corresponding simulation results from random sampling were evaluated via variance-based global sensitivity analysis. The calculated results of the main and total effect indexes show that the test mass rotation angle and the piston effect -lateral significantly influence the TTL coupling noise in the test mass interferometer. The analysis provides a qualitative reference for designing and optimizing space-borne laser interferometry systems.
- space interferometry /
- optical simulation /
- tilt-to-length coupling

HTML全文

Figure 1. Schematic diagram of the simulated optical bench for the test mass interferometer. The left upper corner shows the coordinates of the optical bench, with the detailed coordinates and normal vectors of every optical component in Table 1

下载: 全尺寸图片幻灯片

Figure 2. Schematic illustration of positional factors (a) The offset between the measurement and reference beam (b) The piston effect (c) The test mass shift and rotation

下载: 全尺寸图片幻灯片

Figure 3. The experimental setup schematic. Components included: acousto-optical modulator (AOM), polarizing beam splitter (PBS), half wave plate (λ/2), quarter wave plate (λ/4), beam splitter (BS), stationary mirror (Ref M), fine steering mirror (FSM)

下载: 全尺寸图片幻灯片

Figure 4. Comparison of experimental data and simulation data

下载: 全尺寸图片幻灯片

Figure 5. Histograms of LPS with LPF and AP definitions in 10,000 simulations

下载: 全尺寸图片幻灯片

Figure 6. Visualization of the main effect index (S₁) for the LPF definition

下载: 全尺寸图片幻灯片

Figure 7. Visualization of the total effect index (S_T) for the AP definition

下载: 全尺寸图片幻灯片

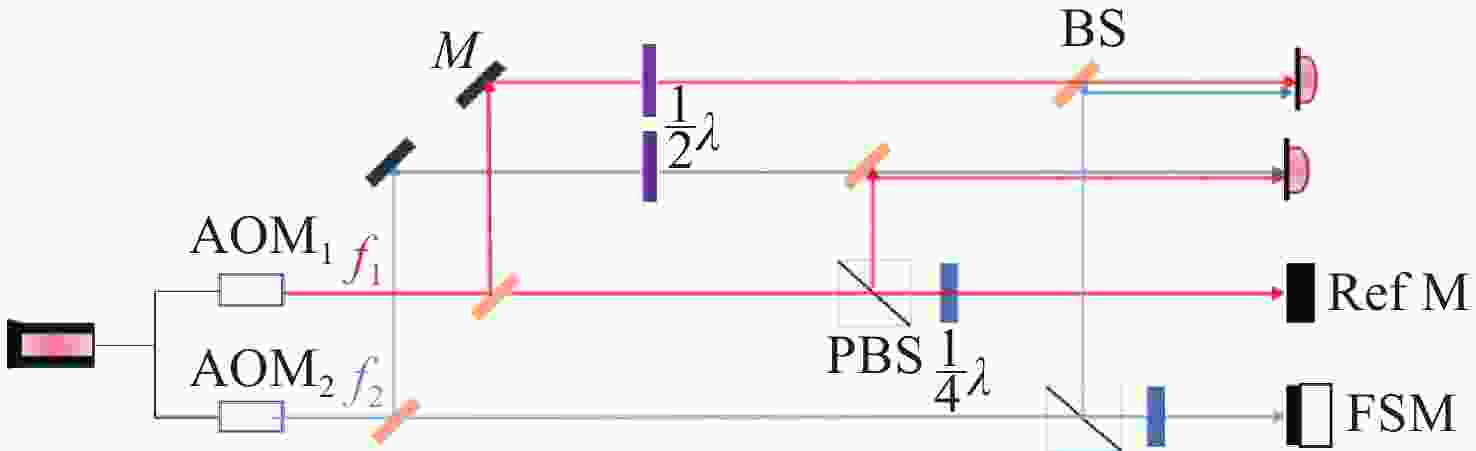
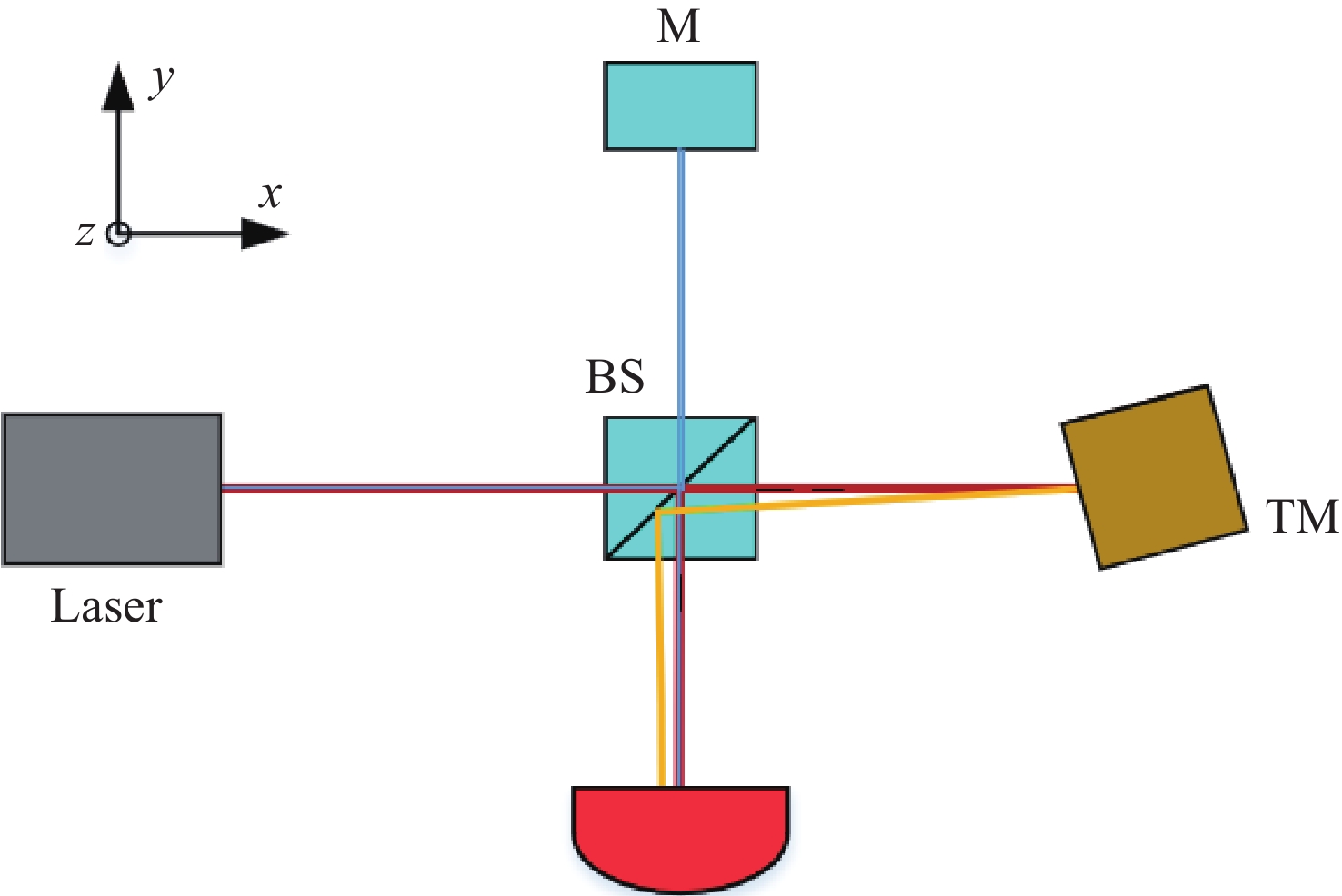
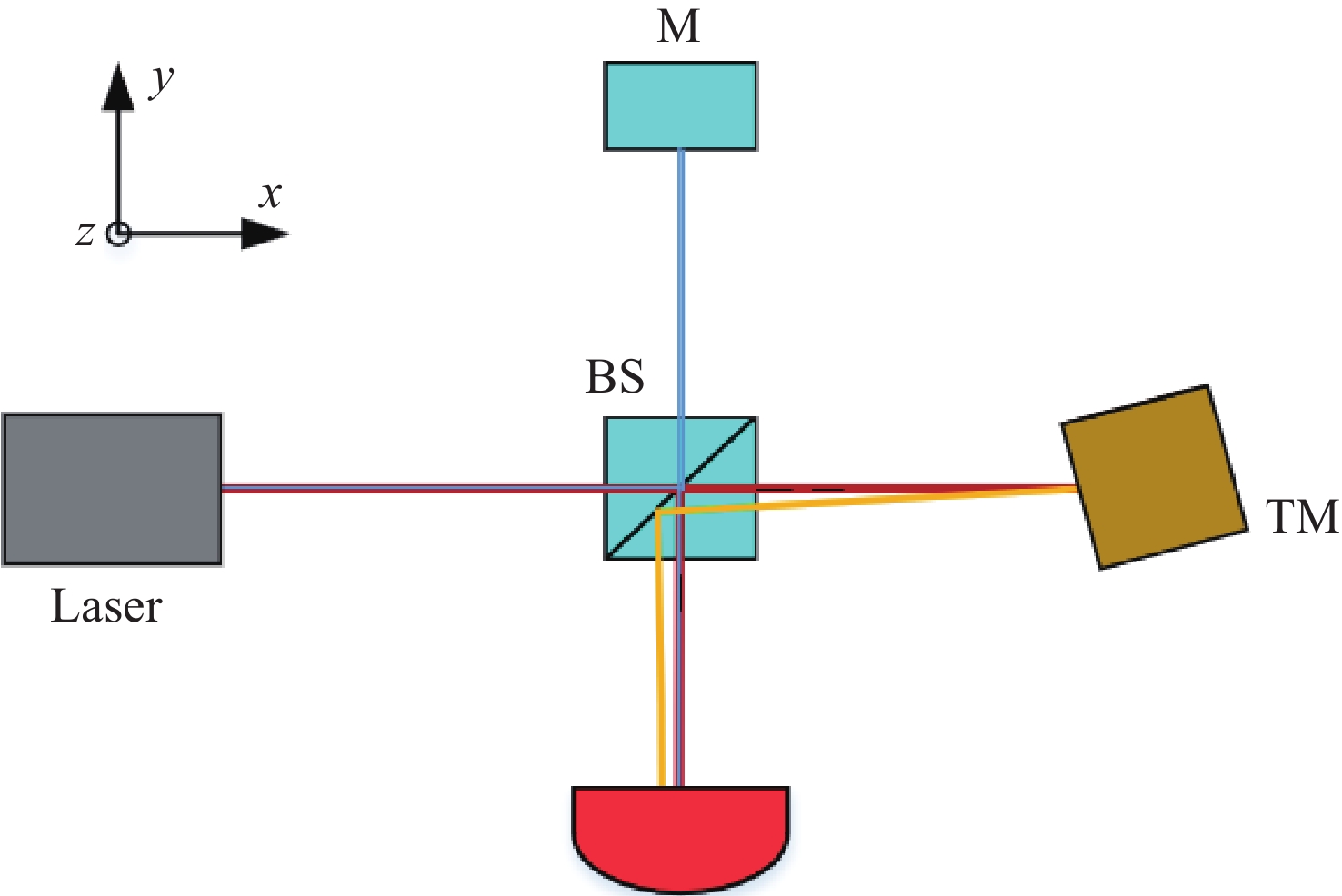
Table 1. The type, position, and orientation of each component in the simulated optical bench used to analyze TTL coupling

Label	Component Name	Center coordinate cm	Normal Vector
Laser	Laser	(0,0,0)	(1,0,0)
BS	Beam splitter	(25,0,0)	(−1,0,0)
M	Mirror	(25,50,0)	(0,−1,0)
TM	Test mass	(50,0,0)	(1,0,0)
QPD	Quadrant photodiode	(25,-25,0)	(0,1,0)

下载: 导出CSV

Table 2. Physical parameters list

Parameter description	Value
Reference beam waist	0.5 mm
Distance from reference beam waist	0
Reference beam frequency	2.8195×10⁸ MHz +120 MHz
Measurement beam waist	0.5 mm
distance from measurement beam waist	0 mm
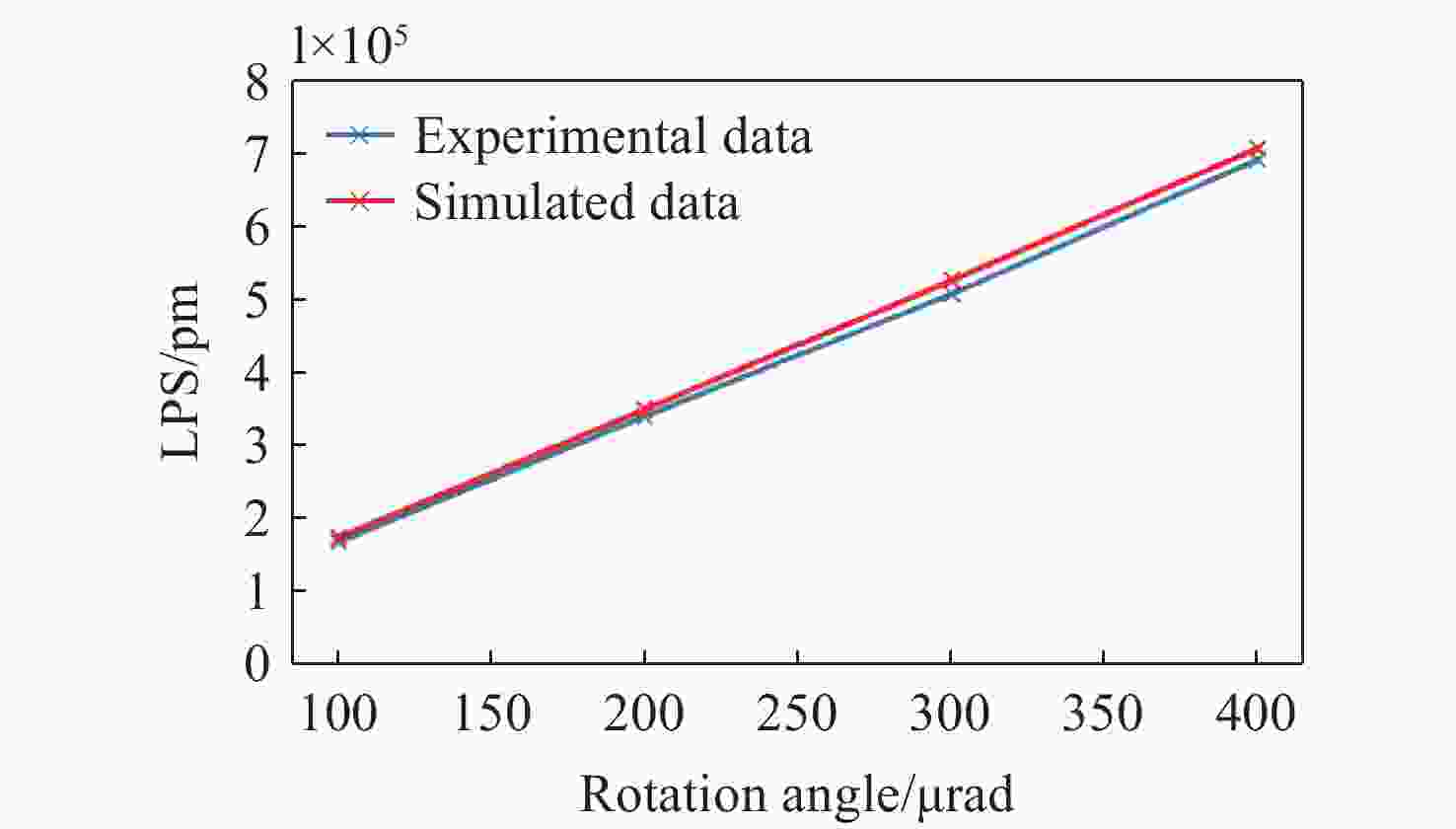
Measurement beam frequency	2.8195×10⁸ MHz + 120 MHz+1.6 MHz
QPD radius	1 cm
QPD slit size	50 µm

下载: 导出CSV

Table 3. Comparison of rotation angle (µrad) and relative error (%)

Rotation Angle (µrad)	Relative Error (%)
100	3.78
200	2.84
300	3.69
400	2.24

下载: 导出CSV

Table 4. Parameter space for multiple factor analysis

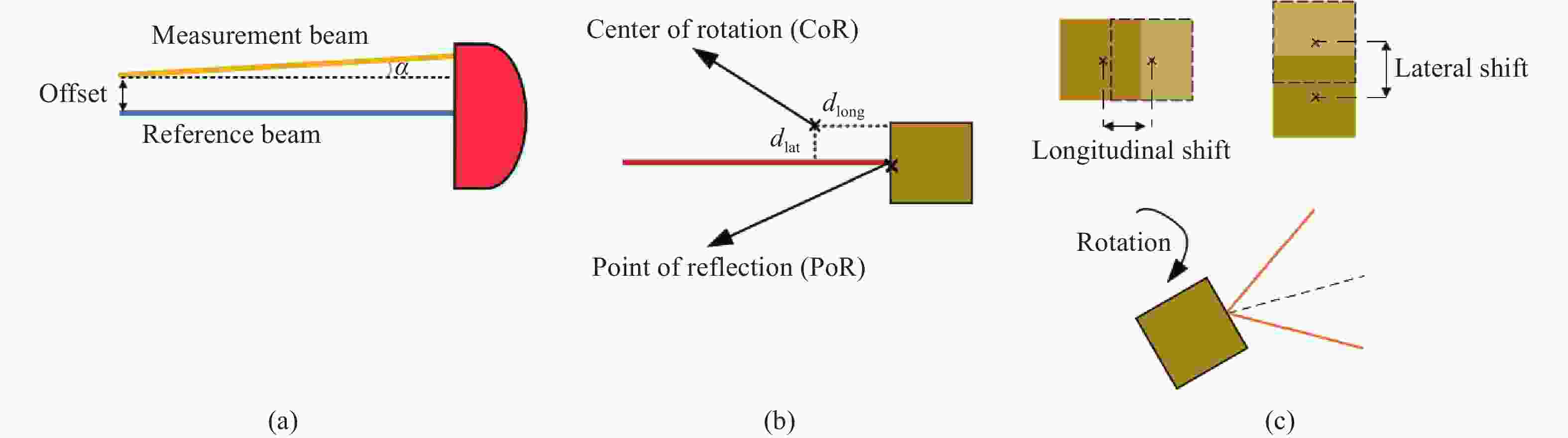
Factors	Parameter	Range
Positional	Beam offset	(0 μm, 100 μm)
	Piston effect (lateral)	(0 mm, 1 mm)
	Piston effect (longitudinal)	(0 mm, 1 mm)
	Test mass lateral shift	(0 μm, 100 μm)
	Test mass longitudinal shift	(0 μm, 100 μm)
	Rotation angle	(0 μrad, 100 μrad)
Beam parameter	Measurement beam waist	(0.5mm, 1 mm)
Beam parameter	The distance from the waist of the measurement beam	(0 mm, 50 mm)
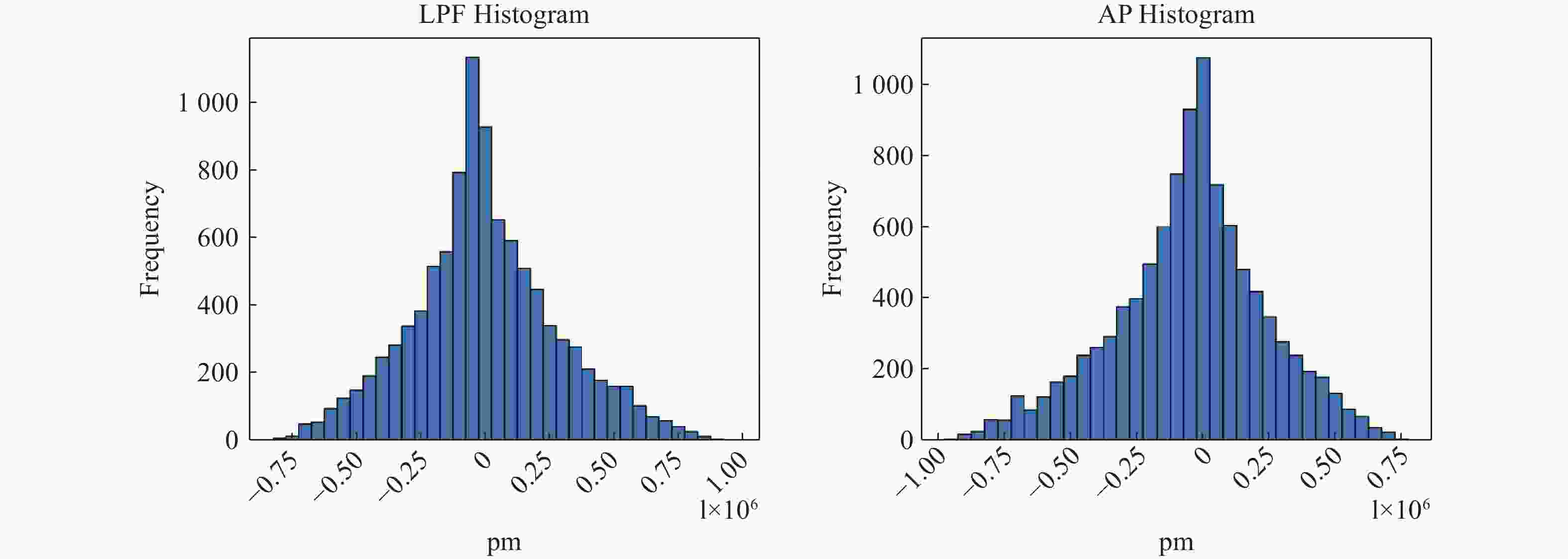
Detector parameter	QPD slit	(0 μm, 100 μm)

下载: 导出CSV

Table 5. Maximum (absolute value) and minimum (absolute value) of the LPS with both LPF and AP in 10,000 simulations.

Signal definition	Maximum	Minimum
LPF	9.6939×10⁵ pm	6.4400 pm
AP	9.1285×10⁵ pm	14.7801 pm

下载: 导出CSV

Table 6. Main effect index S₁ and total effect index S_T for different parameters (LPF definition)

Factors	Parameter	S₁	S_T
Positional	Beam offset	0.00037	0.00023
	Piston effect (lateral)	0.01516	0.21815
	Piston effect (longitudinal)	8.8911×10⁻⁷	5.3478×10⁻⁹
	Test mass lateral shift	−0.00096	0.00217
	Test mass longitudinal shift	−1.0968×10⁻⁷	5.9016×10^-11
	Rotation angle	0.75350	0.99122
Beam parameter	Measurement beam waist	0.00081	0.00240
Beam parameter	Distance from the measurement beam’s waist	5.8822×10⁻⁶	3.1740×10⁻⁷
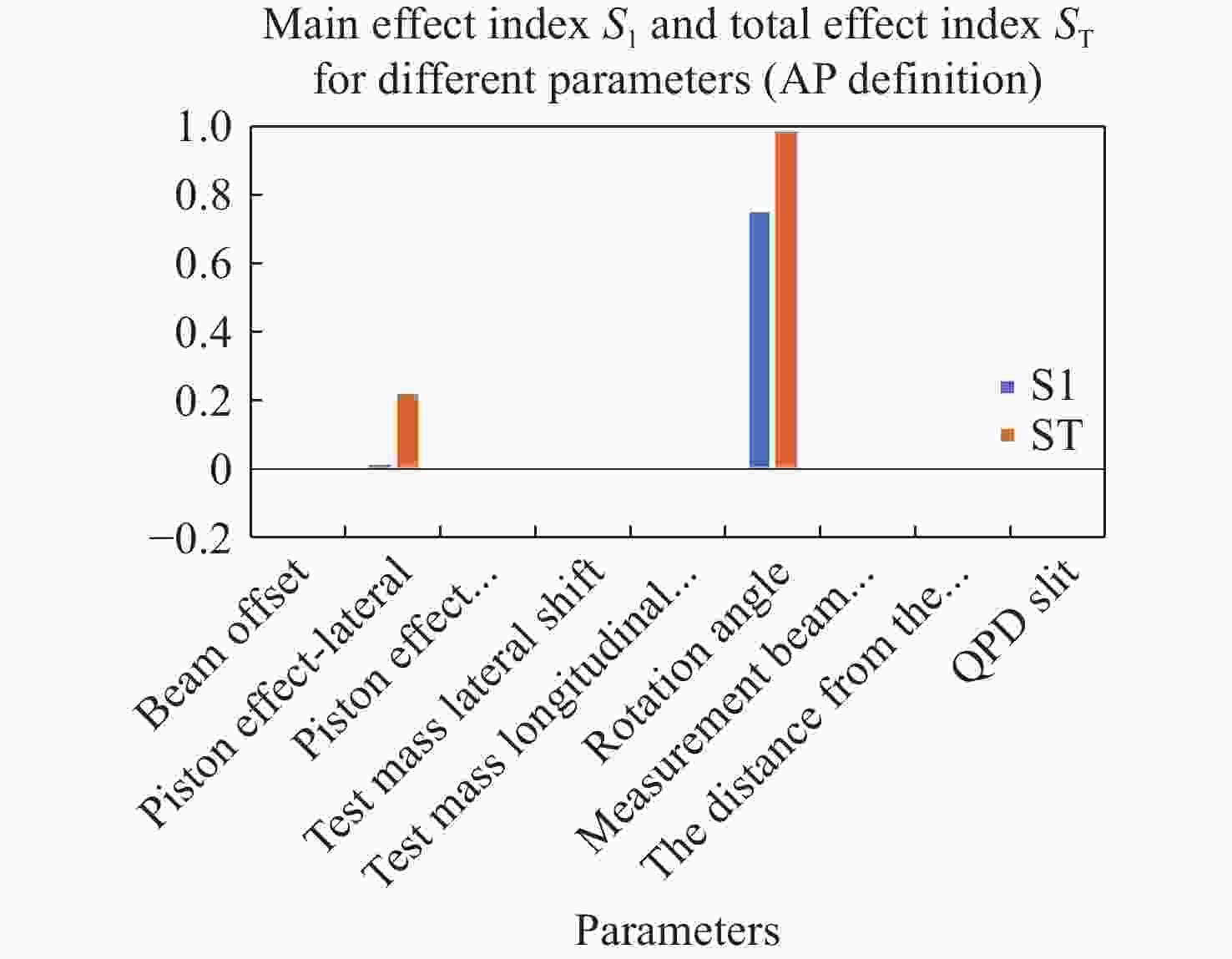
Detector parameter	QPD slit	0.00017	0.00028

下载: 导出CSV

Table 7. Main effect index S₁ and total effect index S_T for different parameters (AP definition)

Factors	Parameter	S₁	S_T
Positional	Beam offset	0.00093	0.00047
	Piston effect (lateral)	0.01409	0.21586
	Piston effect (longitudinal)	1.6607×10⁻⁶	5.29173×10⁻⁹
	Test mass lateral shift	-0.00117	0.00214
	Test mass longitudinal shift	−1.6703×10⁻⁷	7.6198×10^-11
	Rotation angle	0.75988	0.99156
Beam parameter	Measurement beam waist	0.00085	0.00039
Beam parameter	Distance from the measurement beam’s waist	2.1826×10⁻⁶	5.2593×10⁻⁸
Detector parameter	QPD slit	0.00073	0.00035

下载: 导出CSV

参考文献(26)

[1]	AMARO-SEOANE P, AUDLEY H, BABAK S, et al. Laser interferometer space antenna[J]. arXiv preprint arXiv: 1702.00786, 2017. (查阅网上资料, 请核对文献类型及格式) .
[2]	LUO Z R, ZHANG M, JIN G, et al. Introduction of Chinese space-borne gravitational wave detection program “Taiji” and “Taiji-1” satellite mission[J]. Journal of Deep Space Exploration, 2020, 7(1): 3-10.
[3]	LUO J, CHEN L SH, DUAN H Z, et al. TianQin: a space-borne gravitational wave detector[J]. Classical and Quantum Gravity, 2016, 33(3): 035010. doi: 10.1088/0264-9381/33/3/035010
[4]	OTTO M. Time-delay interferometry simulations for the laser interferometer space antenna[D]. Hannover: Gottfried Wilhelm Leibniz Universität Hannover, 2015.
[5]	SCHUSTER S, WANNER G, TRÖBS M, et al. Vanishing tilt-to-length coupling for a singular case in two-beam laser interferometers with Gaussian beams[J]. Applied Optics, 2015, 54(5): 1010-1014. doi: 10.1364/AO.54.001010
[6]	WANG L Y, LI Y Q, CAI R. Noise suppression of tilt-to-length coupling in space laser interferometer[J]. Optics and Precision Engineering, 2021, 29(7): 1491-1498. (in Chinese). doi: 10.37188/OPE.20212907.1491
[7]	CHWALLA M, DANZMANN K, BARRANCO G F, et al. Design and construction of an optical test bed for LISA imaging systems and tilt-to-length coupling[J]. Classical and Quantum Gravity, 2016, 33(24): 245015. doi: 10.1088/0264-9381/33/24/245015
[8]	SCHUSTER S. Tilt-to-length coupling and diffraction aspects in satellite interferometry[D]. Hannover: Gottfried Wilhelm Leibniz Universität Hannover, 2017.
[9]	HARTIG M S, SCHUSTER S, WANNER G. Geometric tilt-to-length coupling in precision interferometry: mechanisms and analytical descriptions[J]. Journal of Optics, 2022, 24(6): 065601. doi: 10.1088/2040-8986/ac675e
[10]	HARTIG M S, SCHUSTER S, HEINZEL G, et al. Non-geometric tilt-to-length coupling in precision interferometry: mechanisms and analytical descriptions[J]. Journal of Optics, 2023, 25(5): 055601. doi: 10.1088/2040-8986/acc3ac
[11]	ZHAO Y, WANG ZH, LI Y P, et al. Method to remove tilt-to-length coupling caused by interference of flat-top beam and Gaussian beam[J]. Applied Sciences, 2019, 9(19): 4112. doi: 10.3390/app9194112
[12]	WANNER G, SCHUSTER S, TRÖBS M, et al. A brief comparison of optical pathlength difference and various definitions for the interferometric phase[J]. Journal of Physics: Conference Series, 2015, 610: 012043. doi: 10.1088/1742-6596/610/1/012043
[13]	SASSO C P, MANA G, MOTTINI S. Coupling of wavefront errors and pointing jitter in the LISA interferometer: misalignment of the interfering wavefronts[J]. Classical and Quantum Gravity, 2018, 35(24): 245002. doi: 10.1088/1361-6382/aaea0f
[14]	ZHAO Y, SHEN J, FANG CH, et al. Tilt-to-length noise coupled by wavefront errors in the interfering beams for the space measurement of gravitational waves[J]. Optics Express, 2020, 28(17): 25545-25561. doi: 10.1364/OE.397097
[15]	ZHAO Y, SHEN J, FANG CH, et al. Far-field optical path noise coupled with the pointing jitter in the space measurement of gravitational waves[J]. Applied Optics, 2021, 60(2): 438-444. doi: 10.1364/AO.405467
[16]	LI J C, LIN H A, LUO J X, et al. Optical design of space gravitational wave detection telescope[J]. Chinese Optics, 2022, 15(4): 761-769. (in Chinese). doi: 10.37188/CO.2022-0018
[17]	SCHUSTER S, TRÖBS M, WANNER G, et al. Experimental demonstration of reduced tilt-to-length coupling by a two-lens imaging system[J]. Optics Express, 2016, 24(10): 10466-10475. doi: 10.1364/OE.24.010466
[18]	WEAVER A J. Investigating limits on gravitational wave detection by laser interferometry using hermite-gauss mode representations of paraxial light propagation[D]. Gainesville: University of Florida, 2021.
[19]	PACZKOWSKI S, GIUSTERI R, HEWITSON M, et al. Postprocessing subtraction of tilt-to-length noise in LISA[J]. Physical Review D, 2022, 106(4): 042005. doi: 10.1103/PhysRevD.106.042005
[20]	HOUBA N, DELCHAMBRE S, ZIEGLER T, et al. Optimal estimation of tilt-to-length noise for spaceborne gravitational-wave observatories[J]. Journal of Guidance, Control, and Dynamics, 2022, 45(6): 1078-1092. doi: 10.2514/1.G006064
[21]	GEORGE D, SANJUAN J, FULDA P, et al. Calculating the precision of tilt-to-length coupling estimation and noise subtraction in LISA using Fisher information[J]. Physical Review D, 2023, 107(2): 022005. doi: 10.1103/PhysRevD.107.022005
[22]	ZHAO M Y, PENG X D, YANG ZH, et al. Preliminary simulation of intersatellite laser interference link for the Taiji program[J]. Journal of Astronomical Telescopes, 2022, 8(3): 038002.
[23]	SOBOL’ I M. On sensitivity estimation for nonlinear mathematical models[J]. Matematicheskoe Modelirovanie, 1990, 2(1): 112-118.
[24]	HOMMA T, SALTELLI A. Importance measures in global sensitivity analysis of nonlinear models[J]. Reliability Engineering & System Safety, 1996, 52(1): 1-17.
[25]	SOBOL’ I M. Global sensitivity indices for nonlinear mathematical models and their Monte Carlo estimates[J]. Mathematics and Computers in Simulation, 2001, 55(1-3): 271-280. doi: 10.1016/S0378-4754(00)00270-6
[26]	SALTELLI A, ANNONI P, AZZINI I, et al. Variance based sensitivity analysis of model output. Design and estimator for the total sensitivity index[J]. Computer Physics Communications, 2010, 181(2): 259-270. doi: 10.1016/j.cpc.2009.09.018